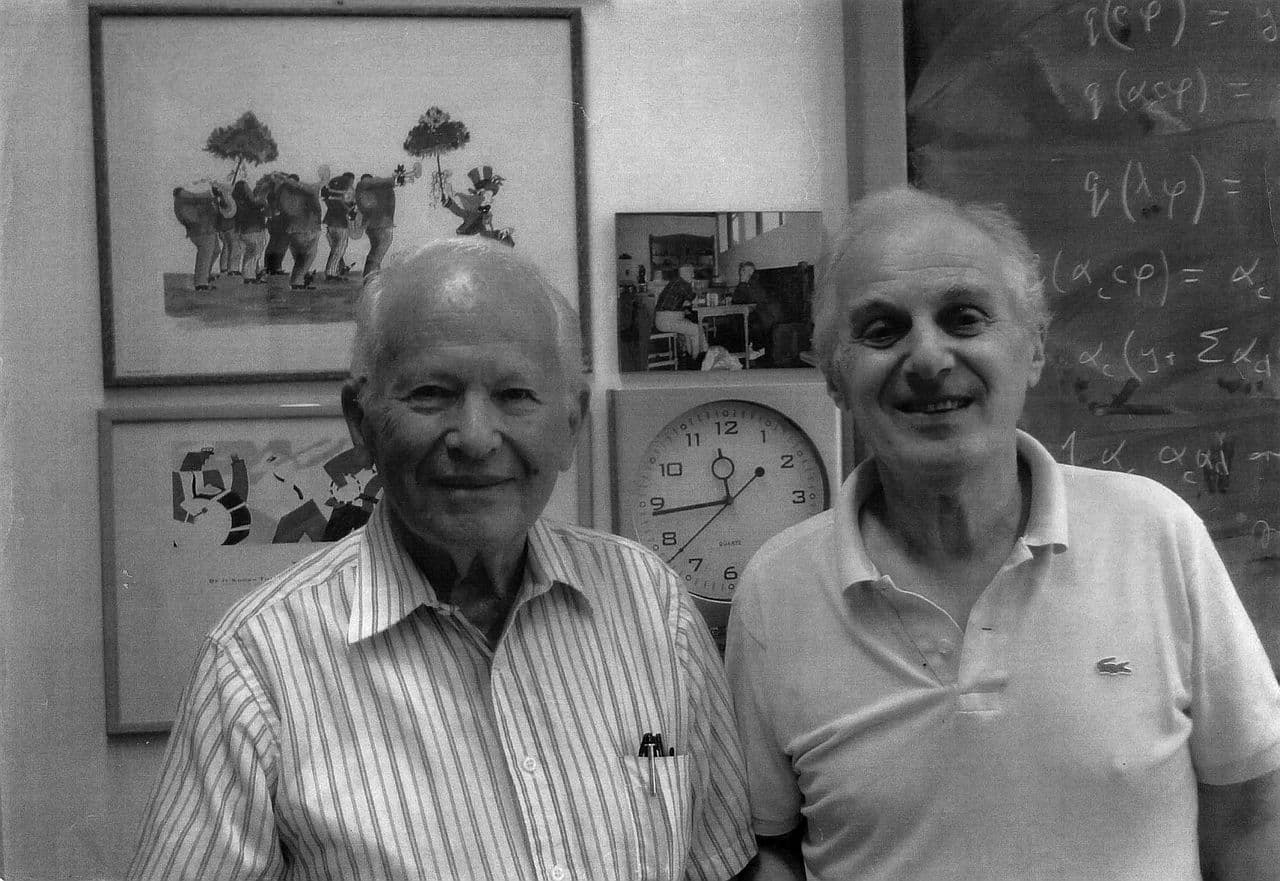My memories with László Fuchs

I will try in these notes to retrace the most important moments I spent with László Fuchs, or at least those which resurface most easily in my memory, and also some moments spent together with his wife Shula and our families.

I met László for the first time in 1972 in Rome, at the Istituto Nazionale di Alta Matematica, where Adalberto Orsatti had organised a conference on Abelian groups. Several mathematicians from the United States attended the conference, including László, who just a few years ago had moved from Budapest to the United States, where he was a professor at Tulane University in New Orleans.

In Rome, Adalberto introduced me to László and asked him if I could go to Tulane for a year to work with him. László’s answer was positive. I was a little excited about the idea, having never been to the United States, and a little apprehensive, being still a novice in research, whereas László was already then considered the foremost expert in Abelian group theory, having published the monograph Abelian Groups in 1958, and his two volumes Infinite Abelian Groups having come out in the early 1970s. Moreover, the twenty-two-year age difference made me see László as a very successful famous mathematician, far above the level of a young researcher at the beginning of his career, as I was then.

In 1972, I was Adalberto Orsatti’s algebra assistant in Ferrara. Three years later I took advantage of a ‘ternata’ in a competition for an assistant professor of analysis post to return to Padua, my home town where I had graduated and where I was to marry Paola in April 1975. I applied for funding from the CNR for a one-year scholarship to spend in the States doing research with Fuchs. The application was accepted, so at the end of October 1975 I left for New Orleans, where Paola, who was then teaching at a middle school, joined me before Christmas. When I arrived at the New Orleans airport, I found László who had come to pick me up and who, fearing I would not recognise him after three years, had a flashy orange Mathematical Reviews issue under his arm.

Of that year spent in New Orleans in the student Rosen House, where we had a small two-room flat as accommodation, and at Gibson Hall, a beautiful neo-Gothic building where Tulane University’s mathematics department was located, Paola and I have the vivid memory of a happy time. The majestic oak trees of Audubon Park near the department filled us with admiration, and visits to the French Quarter and Central Grocery selling Italian delicacies brightened our weekends. László taught a course on Abelian groups to PhD students, whose notes I still keep, filling several notepads. In that course I learnt the main results on totally projective groups, which made me passionate about the theory of Abelian $p$-groups. I then became interested in and studied for many months the existing literature on this theory, hitherto almost completely unknown to me.

One evening we were invited to dinner at László’s house on Laudun Street, where we got to know Shula, who was pregnant with Terry. Paola and I certainly did not think on that occasion that we would become close friends with Shula and László, as in fact happened a few years later.

Thanks to László’s great willingness to help, we tackled together, with him leading and me following, some problems on Abelian groups, so that by the end of the year spent at Tulane I had three published papers with László as co-author. The last of these three papers concerned cotorsion groups, to which I continued to devote myself once I returned to Padua in 1976. So much so that in December 1977, at the second conference organised by Orsatti at the Istituto di Alta Matematica in Rome, I presented the work Cotorsion theories for Abelian groups, later published in 1979 in the conference proceedings, which was to be so successful from 2000 onwards after Göbel and Shelah brought it back into the limelight. Naturally, László attended that conference, which was an opportunity for us to meet Rüdiger Göbel, an Essen mathematician with a background in physics, who would become a close friend of both of us, as well as a prestigious algebraist.

Of the years following our return to Padua in 1976 I have many overlapping memories. Among these is the bridge that was created between Padua and New Orleans, since in those years first Claudia Metelli went to work with László at Tulane, in two different one-year terms, joined one year by Silvana Bazzoni, and then Elisabetta Monari Martinez, all professors at Padua. I remember a nice dinner at Claudia’s house with László and Shula and Tulane algebraist John Dauns. A second group of memories concerns the countless visits that László made to Padua to work with his Paduan colleagues, often bringing with him his family, which had meanwhile grown due to the arrival first of Terry and then of David. For accommodation, I found flats for rent on various occasions. For those who know a little about Padua, they were first located in Via S. Rosa, then in Via XX Settembre, then in Via Crescini (the house that Shula appreciated more than any other) and many times in La Nave, the university’s guest quarters in Portello, a historic Paduan neighbourhood near the mathematics department.

There is an amusing episode concerning the first visit László and Shula made to Padua in 1977, with Terry only a few months old. I had found for them a flat owned by family friends, as I have already mentioned, on Via S. Rosa in the centre of the town. However, I had not thought about Terry, so the first night Shula, not knowing where to put her to sleep, put her in a wardrobe drawer on top of a blanket. The next day I managed to get a camp bed. I also remember that during one of my stays in Padua I took László and Shula for a drive in the Euganean Hills; at one point a group of guinea fowls crossed the road; when Shula asked me what kind of birds they were, I answered that they were special chickens, which amused Shula very much, who then always remembered the special chickens.

An important moment in our scientific collaboration, which I also recalled recently in the lecture I gave in Budapest in June 2024 on the occasion of the Big Five Centenary, which I will discuss later, took place in July 1979. We were with many other mathematicians in Montreal for a summer school organised by the local department of mathematics, which lasted a month and in which László was one of the lecturers. During a picnic on a weekend, László proposed that I begin the systematic study of modules on valuation domains with him, which had been studied up to then by various algebraists, but not in a systematic way. Of this particular episode there remains as a memento a photograph (see Figure ⁠1⁠) that shows, around a picnic table, László with Mario Fiorentini, a recently deceased Roman surveyor who was well known in Italy not only for his mathematical merits, with Adalberto Orsatti and with me.

That was the beginning of a much more intense collaboration than the one we had had until then. We tackled a series of problems together in which László’s great experience and my enthusiasm produced blossoming results and work, in which first Paolo Zanardo and then Silvana Bazzoni were involved. So much so that after only five years, we arranged the knowledge based on the previous work of great algebraists, among whom I cannot fail to mention Irving Kaplansky and Robert Warfield, and on the developments we had achieved in five years, in the first of the two books we wrote together, Modules over Valuation Domains, published in 1985 with Marcel Dekker, Inc.

I already had the fatigue and satisfaction of having published my own book behind me. In fact, in 1980, the monograph Struttura dei $p$-Gruppi Abeliani had come out in the Quaderni dell’Unione Matematica Italiana, and it was perhaps for this reason that László proposed that we write the book on modules on valuation domains together. The preparation of this first book was useful and valuable, allowing me to learn ‘from the inside’ the way László organised his books. We would start with the ‘skeleton,’ i.e., the list of chapters, each divided into its various sections, and for each chapter we would list the articles in the literature that related to the topics to be covered and that we would have to consult or study from scratch. For both this book and the next one, which was much more challenging, we agreed to write half chapters each. However, my English was far from perfect, to put it mildly, so László revised the chapters I had written and put them into good English. Moreover, and not unimportantly, the valuable ‘Notes’ at the end of each chapter were almost always his work, his algebraic knowledge being far superior to mine, while for the list of open problems at the end of the chapter our contributions were almost equal. These dynamics were repeated when we worked on our second book, Modules over Non-Noetherian Domains, published in 2001 by the American Mathematical Society.

I now focus my memories on this second book. I recall that we were in Colorado Springs at the conference organised by Rangaswamy in the summer of 1996, which many of us from Padua attended. One afternoon, after the lectures were over, I found myself with László, who proposed that we write a book together on modules on integral domains, looking almost exclusively at non-Noetherian domains, since existing books on Noetherian domains and their modules numbered in the dozens. I had just come back from work done together with Silvana Bazzoni on modules on particular non-Noetherian domains, which we had called Warfield domains, in honour of the late Robert B. Warfield, Jr., while László had long-standing experience on certain classes of non-Noetherian rings, having worked on them since the late 1940s. Given the positive experience of the previous book, I gladly accepted László’s proposal, warning him, however, that until the end of that year I was still totally absorbed by my position as faculty dean in Padua. So with the new year 1997 we began to think about the new book, first defining its skeleton, consisting of 16 chapters divided into over a hundred sections, which eventually became 126, and then by drawing up the list of papers to be examined; here the professionalism of László, who regularly updated his database of dedicated literature, was decisive.

For three consecutive years, from 1997 to 1999, I spent a week in January in New Orleans as a guest of Shula and László on Laudun Street. We worked many hours a day on the new book, also studying subjects unfamiliar to us. Our laborious and challenging endeavour lasted four years, and the book came out in 2001 with a beautiful dedication written by László for our respective parents. In the preparation of the book, I had an advantage over László, because I could confront myself with Paolo Zanardo and Silvana Bazzoni, who had worked with me for years. Of the two research topics I have studied most in recent years, in fact, the one of finitely generated modules had seen me side by side with Paolo, while the one of non-standard uniserial modules had been investigated with Silvana. Of the latter strand of research, I wrote an article entitled Fascinating modules over valuation domains, based on the conference I held in Budapest in June 2024. In that article I told of the time when with László, during a conference I organised in Udine in 1984, we involved Saharon Shelah in studying the problem of the existence of non-standard uniserial modules, which he solved overnight, and how for many years this problem fascinated some of the most brilliant scholars of rings and modules.

Changing genre of memories, some trips made together with Shula and László come to mind. Two trips I remember with particular pleasure: the first to Port Arthur, Tasmania, during the conference in Hobart that followed the Perth conference in Australia organised by Phil Schultz in 1987; the second to Ireland, at the end of the conference organised in Dublin in 1998 by Brendan Goldsmith.

Port Arthur is a former convict settlement on Tasmania’s Tasman Peninsula. It is about 97 kilometres south-east of the state capital, Hobart, where the second Australian convention of 1987 took place. Shula and László were in Hobart with Terry (11 years old) and David (9 years old), while Paola and I were with our two children Irene (9 years old) and Iacopo (4 years old) (the third, Giuseppe, was not yet born and would later blame us for not taking him with us to Australia). We had rented two small flats in the same building, so communication between the two families was daily, so much so that we celebrated my daughter Irene’s ninth birthday together in our small flat. During the weekend we rented a large family car with a large boot where a couple of children could take turns. We spent a beautiful day visiting the ruins of the prison houses, documenting escape attempts made many years ago by inmates who ended up being eaten by sharks – stories which disturbed and fascinated the little ones.

On the Ireland tour, the Salce line-up included, in addition to Paola and me, Iacopo and Giuseppe, then aged 15 and 9, while the Fuchs line-up included, in addition to Shula and László, our mutual friend Claudia Metelli. We were in two rented cars and toured most of Ireland. I remember that I got a flat tyre on a rough stretch of the road and that László’s help in changing the tyre was providential. I have kept a photo taken by Iacopo as we were changing the tyre, László caught bent over in profile and me, leaning forward, mercilessly taken from the back. The black Cliffs of Mohere, the emerald expanses of the Irish meadows, the stops at local pubs (see Figure ⁠2⁠) and the tasty salmon dinners (which only Iacopo detested) stand out in my memory.

And we come to the new century, which began, as already mentioned, with the release of our second book in 2001. From then on, our collaboration slowed down a lot and was episodic, resulting in five works, four to six years apart. One of our works with Jan Trlifaj on strongly flat modules came out in 2004 [⁠4⁠ L. Fuchs, L. Salce and J. Trlifaj, On strongly flat modules over Matlis domains. In Rings, modules, algebras, and abelian groups, Lecture Notes in Pure and Appl. Math. 236, Dekker, New York, 205–218 (2004) ], a second with Rüdiger Göbel in 2010 on inverse-direct systems of modules [⁠1⁠ L. Fuchs, R. Göbel and L. Salce, On inverse-direct systems of modules. J. Pure Appl. Algebra 214, 322–331 (2010) ], a third with Paolo Zanardo in 2014 on divisibility in cyclically presented modules [⁠5⁠ L. Fuchs, L. Salce and P. Zanardo, Divisibility in cyclically presented modules over integral domains. Houston J. Math. 40, 663–680 (2014) ], a fourth with only László and myself as co-authors in 2018 on almost perfect rings with zero-divisors [⁠3⁠ L. Fuchs and L. Salce, Almost perfect commutative rings. J. Pure Appl. Algebra 222, 4223–4238 (2018) ], and finally our last work on cellular covers with Brendan Goldsmith and Lutz Strüngmann in 2024 [⁠2⁠ L. Fuchs, B. Goldsmith, L. Salce and L. Strüngmann, Cellular covers of divisible uniserial modules over valuation domains. Forum Math. 36, 1613–1629 (2024) ]. Interspersed between these five works, László has published on his own or with other co-authors, after 2001 and to date, another 50 works, many with the Korean Sang Bum Lee, with Rüdiger Göbel, with William Heinzer and Bruce Olberding, and with Kulumani M. Rangaswamy, to name only the most frequent co-authors.

I want to recall how our collaboration came about in the last two works mentioned, the 2018 one on almost perfect rings [⁠3⁠ L. Fuchs and L. Salce, Almost perfect commutative rings. J. Pure Appl. Algebra 222, 4223–4238 (2018) ] and the 2024 one on cellular covers [⁠2⁠ L. Fuchs, B. Goldsmith, L. Salce and L. Strüngmann, Cellular covers of divisible uniserial modules over valuation domains. Forum Math. 36, 1613–1629 (2024) ].

On the occasion of my retirement, Silvana and Paolo, with the help of Riccardo Colpi and Alberto Tonolo, organised a conference in the department on 30 September 2016, at which László, Alberto Facchini, Brendan Goldsmith, Jan Trlifaj and Peter Vámos gave a lecture. László brought me his latest book Abelian Groups, just published by Springer, as a gift. I was admirably astonished at how László had managed, at the age of 90, to produce that comprehensive and weighty book, which updated his two volumes of 1970 and 1973 about 40 years later. The dinner held at the end of that day was attended by many colleagues from the department and many algebraists who had come from various parts of Italy, in addition to the lecturers, and, very welcome, Rangaswamy with his wife Sarah. László, who stayed in Padua with Shula for a few days, came to my studio (see Figure ⁠3⁠) to propose that I help him complete a paper with which he intended to extend the almost perfect domains, which I had studied with Silvana in 2002, to rings with zero-divisors. The work was already well advanced, but several finishing touches were needed, which we worked on together. That was the last time we met Shula, who passed away at Easter 2023.

Speaking of celebrations, I am reminded of when two years earlier, in 2014, László turned 90. A gathering of a few colleagues was organised in New Orleans – in non-Tulane premises – with László’s family, Shula, Terry and David and their children. Rangaswamy and Bruce Olberding gave two lectures on László’s research in commutative algebra, while I spoke about recent results in module theory. At the end, Brendan Goldsmith gave a wonderful talk on the merits and great qualities of László, both as a mathematician and as a friend and point of reference for the large community of algebraists who had worked on Abelian groups and modules. It was an event with few participants, but very intense, which I was also happy to attend with Paola.

The history of the eight-handed work on cellular covers is particularly curious. In January 2023, our department organised a conference for the retirement of Alberto Facchini. At the final dinner I was at the table with Paolo and Paola Zanardo, Sylvia and Roger Wiegand, Patrizia Longobardi and Ann and Brendan Goldsmith. Towards the end of the dinner, Brendan came up next to me and suggested that I look at some notes by László, Lutz Strüngmann and him on cellular covers for modules on valuation domains. Here is the remark that appears at the end of the introduction to the eight-handed paper published in 2024, Cellular covers of divisible uniserial modules over valuation domains [⁠2⁠ L. Fuchs, B. Goldsmith, L. Salce and L. Strüngmann, Cellular covers of divisible uniserial modules over valuation domains. Forum Math. 36, 1613–1629 (2024) ].

Remark. This paper has had a long gestation period: it started with an unpublished preliminary note of 2011 in which László Fuchs prompted his co-authors to continue to develop the theory of cellular covers for divisible modules over valuation domains. Unfortunately, the note arrived at a time when Rüdiger Göbel was ill and unable to participate in the project. With his untimely death in July 2014, the note remained untouched until 2019 when two of the current authors [Brendan and Lutz – ed.] revived the project and made good progress. They were subsequently joined by Luigi Salce, who succeeded in bringing the project to a partial conclusion. Finally, the ninety-nine-year-old László Fuchs reorganised the material, adding more insights and results, and gave his final touch to the paper. We are delighted to dedicate this work to the memory of our beloved late friend, Rüdiger Göbel, pioneer in our present topic: the algebraic theory of cellular covers, and co-author of many joint papers with each of us.

The work done with Brendan and Lutz is perhaps not the last of the work with co-authors László and me, as that work left a tail with interesting open issues that we are studying together. We will see what will come out of it.

I go now with my memory to my last meeting with László, which took place, as mentioned earlier, in Budapest on 4–5 June 2024. The occasion was the so-called Big Five Meeting, which this special year was called the Big Five Centenary. Traditionally, this event celebrated five great Hungarian mathematicians each ten years, all born in 1924. This year was therefore the centenary, but only László was present, the other four mathematicians having already passed away. As always, the day was organised by the Hungarian Academy of Sciences and the Alfréd Rényi Institute of Mathematics.⁠¹⁠https://mta.hu/english/big-five-100-video-of-the-international-conference-celebrating-the-100th-anniversary-of-the-birth-of-five-legendary-mathematicians-113767 During the ceremony, the president of the Academy presented László with the János Arany Lifetime Achievement Award, the most prestigious academic honour that the Hungarian Academy of Sciences bestows on Hungarian scientists who do not live in Hungary. During those two days, besides enjoying the excellent Hungarian hospitality, I got to spend some time with László and with Terry and David who had accompanied him from Atlanta. László gave a beautiful thank-you speech at the end of the day, which reminded me of the speeches he had given on several occasions at the conclusion of the many conferences on Abelian groups and modules held over more than 50 years.

To conclude, it seems appropriate to add one last note.

In an article that recently appeared in the EMS Magazine, entitled Are old mathematicians useless? [⁠7⁠ A. Pietsch, Are old mathematicians useless? Eur. Math. Soc. Mag. 131, 40–42 (2024) ], Albrecht Pietsch reports the following statements made in G. H. Hardy’s famous book A mathematician’s apology [⁠6⁠ G. H. Hardy, A mathematician’s apology. (With a foreword by C. P. Snow.) Canto, Cambridge University Press, Cambridge (1992) ].

“I do not know an instance of a major mathematical advance initiated by a man past fifty. (…) A mathematician may still be competent enough at sixty, but it is useless to expect him to have original ideas.”

Disagreeing with Hardy, Pietsch comments as follows:

“In the preceding statements Hardy uses two bounds, ‘fifty’ and ‘sixty.’ In my opinion, this difference is inessential, since any age limit should be avoided.”

In support of his opinion, Albrecht Pietsch brings the example of ten great mathematicians who produced mathematical advances and original ideas well after “fifty,” starting with Henri Poincaré and ending with Yitang Zhang. Of these ten, only Henri Cartan and Eugenio Calabi made it to over one hundred years old and only four were born before 1924. To these examples one can therefore with good reason add László Fuchs.

Luigi Salce (born in 1946 in Padua) is professor emeritus of algebra of the University of Padua, where he worked since 1984 as full professor of geometry and algebra. He retired in 2016. He is author of more than 100 papers in several areas of algebra, of two books on matrix theory, of a book on Abelian groups and co-author of two monographs on module theory. He gave lectures in several European countries, in the United States, in China, and in Australia. salce@math.unipd.it

1. ¹

   https://mta.hu/english/big-five-100-video-of-the-international-conference-celebrating-the-100th-anniversary-of-the-birth-of-five-legendary-mathematicians-113767

References

1. L. Fuchs, R. Göbel and L. Salce, On inverse-direct systems of modules. J. Pure Appl. Algebra 214, 322–331 (2010)
2. L. Fuchs, B. Goldsmith, L. Salce and L. Strüngmann, Cellular covers of divisible uniserial modules over valuation domains. Forum Math. 36, 1613–1629 (2024)
3. L. Fuchs and L. Salce, Almost perfect commutative rings. J. Pure Appl. Algebra 222, 4223–4238 (2018)
4. L. Fuchs, L. Salce and J. Trlifaj, On strongly flat modules over Matlis domains. In Rings, modules, algebras, and abelian groups, Lecture Notes in Pure and Appl. Math. 236, Dekker, New York, 205–218 (2004)
5. L. Fuchs, L. Salce and P. Zanardo, Divisibility in cyclically presented modules over integral domains. Houston J. Math. 40, 663–680 (2014)
6. G. H. Hardy, A mathematician’s apology. (With a foreword by C. P. Snow.) Canto, Cambridge University Press, Cambridge (1992)
7. A. Pietsch, Are old mathematicians useless? Eur. Math. Soc. Mag. 131, 40–42 (2024)