Recent progress on the problem of soliton resolution

Jacek Jendrej

doi:10.4171/mag/237

Dispersive partial differential equations are evolution equations whose solutions decay in large time due to the fact that various frequencies propagate with distinct velocities. In some cases, there exist special solutions called solitons, which do not change their shape as time passes. The soliton resolution conjecture predicts that solitons are the only obstruction to the decay of solutions. More precisely, every solution eventually decomposes into a superposition of solitons and a decaying term called radiation. We discuss the conjecture in the context of the wave maps equation, which is the analog of the wave equation for sphere-valued maps.⁠¹⁠This note is based on the talk given by the author at the 9th European Congress of Mathematics.

1 The phenomenon of dispersion

This section is devoted to standard introductory material. For a comprehensive introduction to the topic, the reader can consult for instance [⁠27⁠ F. Linares and G. Ponce, Introduction to nonlinear dispersive equations. Second ed., Universitext, Springer, New York (2015) , ⁠44⁠ G. B. Whitham, Linear and nonlinear waves. Pure and Applied Mathematics, John Wiley & Sons, New York (1999) ].

1.1 The wave equation

Consider the wave equation in dimension $1 + 2$ ,

c^{- 2} \partial_{t}^{2} ψ (t, x_{1}, x_{2}) = \partial_{x_{1}}^{2} ψ (t, x_{1}, x_{2}) + \partial_{x_{2}}^{2} ψ (t, x_{1}, x_{2}),

where $(t, x_{1}, x_{2}) \in R^{1 + 2}$ . The positive number $c > 0$ is the wave speed. Let us assume for simplicity that $ψ$ is real-valued, but it could just as well be vector-valued. We will always write $R^{1 + 2}$ instead of $R^{3}$ in order to stress that one deals with one time dimension and two space dimensions. Equation (⁠W⁠) is equivalent to requiring that $ψ$ is a critical point of the Lagrangian

L (ψ) : = \frac{1}{2} \int_{R^{1 + 2}} (c^{- 2} (\partial_{t} ψ)^{2} - (\partial_{x_{1}} ψ)^{2} - (\partial_{x_{2}} ψ)^{2}) .

The precise meaning of this assertion is the following. Let $ζ : R^{1 + 2} \to R$ be a smooth compactly supported function and $ψ_{ε} : = ψ + εζ$ , where $ε$ is a small real number. We then have

\frac{d}{d ε}_{ε = 0} L (ψ_{ε}) = \int_{R^{1 + 2}} (c^{- 2} \partial_{t} ψ \partial_{t} ζ - \partial_{x_{1}} ψ \partial_{x_{1}} ζ - \partial_{x_{2}} ψ \partial_{x_{2}} ζ)

= - \int_{R^{1 + 2}} ζ (c^{- 2} \partial_{t}^{2} ψ - \partial_{x_{1}}^{2} ψ - \partial_{x_{2}}^{2} ψ),

where the last step is integration by parts. The left-hand side can be interpreted as the directional derivative of $L$ at $ψ$ in the direction $ζ$ . Hence, we see that all the directional derivatives vanish if and only if $ψ$ satisfies (⁠W⁠).

Equation (⁠W⁠) appears in many physical contexts, the most familiar being the evolution in time of a small disturbance of the membrane of a drum. It should be understood that the membrane extends to infinity and occupies the whole horizontal plane, and $ψ (t, x_{1}, x_{2})$ is the vertical displacement at time $t$ of the element of the membrane whose horizontal coordinates are $(x_{1}, x_{2})$ .

Recalling that the Lagrangian density is the difference of the kinetic and the potential energy densities, from the form of (⁠1⁠) we find that the total energy is given by

E (ψ) : = E_{kinetic} + E_{potential}

= \frac{1}{2} \int_{R^{2}} c^{- 2} (\partial_{t} ψ)^{2} + \frac{1}{2} \int ((\partial_{x_{1}} ψ)^{2} + (\partial_{x_{2}} ψ)^{2}),

and is a conservation law (a quantity independent of time).

Remark 1.1. By an appropriate choice of units, one can assume that $c = - 1$ , which we will always do in the sequel. We will also write

x : = (x_{1}, x_{2}), \nabla : = (\partial_{x_{1}}, \partial_{x_{2}}),

Δ : = \partial_{x_{1}}^{2} + \partial_{x_{2}}^{2}, d x : = d x_{1} d x_{2} .

Mechanical intuition suggests that in order to determine the evolution in time of the disturbance of the membrane we need to specify the initial conditions consisting of the initial positions $ψ (0, x)$ and the initial velocities $\partial_{t} ψ (0, x)$ of all the elements of the drum. One can indeed prove that for any such initial conditions, equation (⁠W⁠) has a unique solution for all time, which moreover depends continuously on the initial conditions (in an appropriate sense that we will not make precise here), which is referred to as global well-posedness.

Having once more recourse to the intuition from mechanics, we can expect that, if the membrane is initially perturbed only in a bounded region and flat elsewhere, then this disturbance will propagate in various directions, resulting in a decay of its amplitude, namely

t \to \infty lim x \in R^{2} sup ∣ ψ (t, x) ∣ = 0,

which is referred to as radiative behavior.

1.2 A few generalities on linear dispersive PDEs

Since it is hard to give a rigorous definition of a linear dispersive PDE which would cover all the interesting cases, we limit ourselves to the following heuristic definition.

Definition 1.2. A linear PDE is called dispersive if

it is an evolution equation: it involves the time variable $t$ and the space variable $x$ ,
various frequencies propagate with distinct velocities.

Examples of linear dispersive PDEs include:

the wave equation (⁠W⁠) and its analogs in higher space dimensions,
the Schrödinger equation,
the Klein–Gordon equation,

but the list could be made longer. All these examples are time-reversible and have a conserved energy, and yet smooth localized initial conditions lead to radiative behavior as $t \to \infty$ .

Remark 1.3. Radiative behavior crucially depends on the fact that the spatial domain is the whole Euclidean space (or in any case that it is unbounded).

Remark 1.4. In some sense, for a linear dispersive PDE, the trivial solution ${ψ \equiv 0}$ is the global attractor of the flow.

2 The nonlinear setting: dispersion, solitons, soliton resolution

For a comprehensive introduction to the topic of this section, the reader can consult the monographs [⁠42⁠ T. Tao, Nonlinear dispersive equations: Local and global analysis. CBMS Reg. Conf. Ser. Math. 106, American Mathematical Society, Providence, RI (2006) ] and [⁠36⁠ J. Shatah and M. Struwe, Geometric wave equations. Courant Lect. Notes Math. 2, New York University, Courant Institute of Mathematical Sciences, New York; American Mathematical Society, Providence, RI (1998) ].

2.1 Wave maps

Wave maps are nonlinear, geometric analogs of linear waves in the case of maps taking values in a Riemannian manifold, rather than in a Euclidean space. We consider here wave maps $Ψ : R^{1 + 2} \to S^{2} \subset R^{3}$ . In classical mechanics, a constrained mechanical system is obtained from the same Lagrangian as for the ambient system, see [⁠1⁠ V. I. Arnol’d, Mathematical methods of classical mechanics. Second ed., Grad. Texts in Math. 60, Springer, New York (1989) , Chapter 4]. Following this principle and recalling (⁠1⁠), we say that a map $Ψ : R^{1 + 2} \to S^{2} \subset R^{3}$ is a wave map if it is a critical point of the Lagrangian

L (Ψ) = \frac{1}{2} \int_{R^{1 + 2}} (∣ \partial_{t} Ψ ∣^{2} - ∣ \partial_{x_{1}} Ψ ∣^{2} - ∣ \partial_{x_{2}} Ψ ∣^{2}),

where $∣ \cdot ∣$ denotes the Euclidean norm in $R^{3}$ . Similarly, as in Section ⁠1.1⁠, we can consider $Ψ_{ε} = Ψ + εZ$ , where $Z : R^{1 + 2} \to R^{3}$ is smooth and compactly supported. In order not to violate at main order the condition that $Ψ_{ε}$ takes values in $S^{2}$ , it is necessary and sufficient to require that

Z (t, x) ⊥ Ψ (t, x) for all (t, x) .

As in Section ⁠1.1⁠, we have

\frac{d}{d ε}_{ε = 0} L (Ψ_{ε}) = - \int_{R^{1 + 2}} Z \cdot (\partial_{t}^{2} Ψ - ΔΨ) .

This quantity vanishes for all $Z$ satisfying (⁠8⁠) if and only if

\partial_{t}^{2} Ψ (t, x) - ΔΨ (t, x) = μ (t, x) Ψ (t, x)

for some $μ (t, x) \in R$ . Differentiating twice the identity $Ψ \cdot Ψ = 1$ , we obtain

Ψ \cdot (\partial_{t}^{2} Ψ - ΔΨ) = - ∣ \partial_{t} Ψ ∣^{2} + ∣ \partial_{x_{1}} Ψ ∣^{2} + ∣ \partial_{x_{2}} Ψ ∣^{2},

so we can write the wave map equation $R^{1 + 2} \to S^{2}$ as

\partial_{t}^{2} Ψ - ΔΨ = - (∣ \partial_{t} Ψ ∣^{2} - ∣ \partial_{x_{1}} Ψ ∣^{2} - ∣ \partial_{x_{2}} Ψ ∣^{2}) Ψ.

Similarly, as in the linear case, the total energy

E (Ψ) : = \int_{R^{2}} (\frac{1}{2} ∣ \partial_{t} Ψ ∣^{2} + \frac{1}{2} ∣ \partial_{x_{1}} Ψ ∣^{2} + \frac{1}{2} ∣ \partial_{x_{2}} Ψ ∣^{2})

is a conservation law for wave maps.

Equation (⁠WM⁠) has trivial constant in space-time solutions $Ψ (t, x) = ω_{0} \in S^{2}$ . If we linearize around such a solution, that is, if we write $Ψ = ω_{0} + ε Φ$ with $ε ≪ 1$ and plug into (⁠WM⁠), at main order we obtain $\partial_{t}^{2} Φ - ΔΦ = 0$ ; thus, each component of $Φ$ satisfies the wave equation (⁠W⁠), which indicates that small perturbations of a constant solution should exhibit a radiative behavior, so that the whole wave map should converge to a constant. It was proved in the works of Tataru [⁠43⁠ D. Tataru, On global existence and scattering for the wave maps equation. Amer. J. Math. 123, 37–77 (2001) ] and Tao [⁠41⁠ T. Tao, Global regularity of wave maps. II. Small energy in two dimensions. Comm. Math. Phys. 224, 443–544 (2001) ] that, in an appropriate sense, this is indeed the case.

For the study of the long-time behavior of solutions of (⁠WM⁠), criticality is an important (and helpful) property. Let $λ > 0$ and consider

Ψ_{λ} (t, x) : = Ψ (t / λ, x / λ) .

It is clear from (⁠WM⁠) (or from the Lagrangian) that $Ψ$ is a wave map if and only if $Ψ_{λ}$ is a wave map. Moreover,

E (Ψ_{λ}) = E (Ψ) .

For this reason, equation (⁠WM⁠) is called energy critical, and its solutions critical wave maps.

Remark 2.1. In general, a problem is subcritical if it becomes a “small data problem” when rescaling (zooming) to a small region. It is called supercritical if such a zoom makes it large. It is called critical if the size of the data remains unchanged.

2.2 Harmonic maps

One might wonder if every, not necessarily small, solution of (⁠WM⁠) has radiative behavior. The answer is “no” for a simple reason: there exist non-trivial static (time-independent) solutions. Namely, inserting $Ψ (t, x) = ω (x)$ into (⁠WM⁠), we obtain the critical harmonic map equation:

- Δ ω = ∣ \nabla ω ∣^{2} ω, ω : R^{2} \to S^{2} \subset R^{3} .

Its solutions are called harmonic maps $R^{2} \to S^{2}$ . It was proved by Eells and Wood [⁠13⁠ J. Eells and J. C. Wood, Restrictions on harmonic maps of surfaces. Topology 15, 263–266 (1976) ], and Hélein [⁠17⁠ F. Hélein, Harmonic maps, conservation laws and moving frames. Second ed., Cambridge Tracts in Math. 150, Cambridge University Press, Cambridge (2002) ] that harmonic maps of finite energy correspond to rational functions $S^{2} \to S^{2}$ and their complex conjugates (we identify $R^{2}$ with $S^{2}$ using the stereographic projection).

Remark 2.2. It was proved by Krieger, Schlag and Tataru [⁠26⁠ J. Krieger, W. Schlag and D. Tataru, Renormalization and blow up for charge one equivariant critical wave maps. Invent. Math. 171, 543–615 (2008) ], Rodnianski and Sterbenz [⁠32⁠ I. Rodnianski and J. Sterbenz, On the formation of singularities in the critical O⁢(3)σ-model. Ann. of Math. (2) 172, 187–242 (2010) ], and Raphaël and Rodnianski [⁠31⁠ P. Raphaël and I. Rodnianski, Stable blow up dynamics for the critical co-rotational wave maps and equivariant Yang–Mills problems. Publ. Math. Inst. Hautes Études Sci. 115, 1–122 (2012) ], that solutions of large energy can even cease to exist in finite time. Equation (⁠WM⁠) is thus locally well-posed, but not globally well-posed.

2.3 A few generalities on nonlinear dispersive PDEs

A nonlinear PDE is called dispersive if it is related to a linear dispersive PDE. Most frequently, “related” means “obtained through linearization around trivial solutions,” like in the case of wave maps discussed above.

Nonlinear dispersive PDEs appear frequently in physics, for example in the study of water waves and nonlinear optics, see [⁠44⁠ G. B. Whitham, Linear and nonlinear waves. Pure and Applied Mathematics, John Wiley & Sons, New York (1999) , Chapters 12, 13, 16, 17]. Typical examples are Hamiltonian systems, which, in particular are time-reversible and have a conserved energy.

One is often interested in the dynamical behavior of solutions of a given nonlinear PDE, by which we mean their asymptotic description as time becomes large (for solutions defined for all time; if they are not, one studies the limit as the time tends to the maximal time of existence of the solution). Among the most common questions of this type is the problem of stability, which can be formulated as follows.

Problem. Do small solutions of a nonlinear dispersive PDE exhibit radiative behavior? In other words, does the flow restricted to small solutions have a trivial attractor, like in the linear case (see Remark ⁠1.4⁠)?

The intuitive reasoning is that small solutions should behave in the same way as the solutions of the linearized problem, which have radiative behavior as we saw in Section ⁠1.2⁠.

2.4 Solitons and soliton resolution

The notion of a soliton is somewhat controversial, see [⁠28⁠ N. Manton and P. Sutcliffe, Topological solitons. Cambridge Monogr. Math. Phys., Cambridge University Press, Cambridge (2004) , Section 1.5]. We adopt the following definition.

Definition 2.3. A soliton is a solution of an evolution PDE which does not change its shape in the course of time (it can, however, change its position).

Harmonic maps from Section ⁠2.2⁠ are examples of solitons for (⁠WM⁠). Solitons moving at constant velocity can be obtained using the Lorentz invariance of (⁠WM⁠).

Solitons do not exhibit radiative behavior. The problem of soliton resolution is to prove that they are the only obstruction to radiative behavior. However, it would be too naive to expect that every solution is either radiative or a soliton. Rather, one expects that every solution eventually decomposes into a superposition of solitons which interact sufficiently weakly (for example, they could travel with distinct velocities). Such superpositions are called multisoliton configurations.

Problem (Soliton resolution conjecture). For a given nonlinear dispersive PDE, does every solution converge in large time to the sum of a multisoliton and a radiative term? In other words, does the flow have a simple global attractor related to multisoliton configurations?

The soliton resolution is inspired by

numerical simulations, see Fermi, Pasta and Ulam [⁠14⁠ E. Fermi, J. Pasta and S. Ulam, Studies of nonlinear problems. Technical Report LA-1940, Los Alamos Scientific Laboratory, CA (1955) ], Zabusky and Kruskal [⁠45⁠ N. J. Zabusky and M. D. Kruskal, Interaction of ”solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965) ],
the theory of completely integrable systems, see Segur and Ablowitz [⁠34⁠ H. Segur and M. J. Ablowitz, Asymptotic solutions and conservation laws for the nonlinear Schrödinger equation. I. J. Math. Phys. 17, 710–713 (1976) ], Eckhaus and Schuur [⁠12⁠ W. Eckhaus and P. Schuur, The emergence of solitons of the Korteweg–de Vries equation from arbitrary initial conditions. Math. Methods Appl. Sci. 5, 97–116 (1983) ],
analogous elliptic and parabolic problems (bubbling), see Sacks and Uhlenbeck [⁠33⁠ J. Sacks and K. Uhlenbeck, The existence of minimal immersions of 2-spheres. Ann. of Math. (2) 113, 1–24 (1981) ], Struwe [⁠39⁠ M. Struwe, On the evolution of harmonic mappings of Riemannian surfaces. Comment. Math. Helv. 60, 558–581 (1985) ].

Remark 2.4. Even with such a vague formulation, the soliton resolution is not expected to hold for all nonlinear dispersive PDEs. For example, the sine-Gordon equation has so-called breather solutions, which do not fall into the regime of soliton resolution.

Remark 2.5. Strictly speaking, soliton resolution only provides an “upper bound” on the global attractor, in the sense that it does not say anything on the types of multisoliton configurations which can be realized by the evolution.

Our main goal is to provide an example of “natural” nonlinear dispersive PDEs for which we can prove soliton resolution. Even though we cannot provide a full description of the global attractor, we will see that it contains configurations consisting of more than one soliton.

3 Soliton resolution for equivariant energy-critical wave maps

3.1 Equivariant maps

The governing PDE will be obtained from (⁠WM⁠) by restricting the flow to a certain subclass of all the maps $R^{2} \to S^{2}$ which is preserved by the flow. They are called equivariant maps and are defined in the following way. We fix $k \in {1, 2, \dots}$ and consider maps of the form

Ψ (t, r cos θ, r sin θ)

= (sin (ψ (t, r)) cos (k θ), sin (ψ (t, r)) sin (k θ), cos (ψ (t, r))),

where $r > 0$ and $ψ (t, r) \in R$ . Plugging this expression into (⁠WM⁠), we find the scalar equation

\partial_{t}^{2} ψ (t, r) - \partial_{r}^{2} ψ (t, r) - \frac{1}{r} \partial_{r} ψ (t, r) + \frac{k ^{2}}{2 r ^{2}} sin (2 ψ (t, r)) = 0,

where $r > 0$ is the radial coordinate. Note that $ψ$ and $ψ + 2 π ℓ$ represent the same map $Ψ$ for any $ℓ \in Z$ .

Remark 3.1. In the non-geometric context, it is common to consider spherically symmetric solutions. Equivariant solutions are analogous objects in the geometric setting of (⁠WM⁠). More generally, whenever symmetries of a given equation lead to invariance of a certain class of states, it is a well-known technique to study the restriction of the system to this subclass.

One can check that under the substitution (⁠15⁠), the Lagrangian (⁠7⁠) becomes

L : = π \iint ((\partial_{t} ψ)^{2} - (\partial_{r} ψ)^{2} - \frac{k ^{2} sin ( ψ ) ^{2}}{r ^{2}}) r d r d t .

Its critical points are thus $k$ -equivariant wave maps, a fact that can easily be checked directly. The kinetic energy and the potential energy are

E_{kinetic} : = π \int_{0}^{\infty} (\partial_{t} ψ)^{2} r d r,

E_{potential} : = π \int_{0}^{\infty} ((\partial_{r} ψ)^{2} + \frac{k ^{2} sin ( ψ ) ^{2}}{r ^{2}}) r d r .

Their sum is the total energy, and it is a conserved quantity.

We always consider strong solutions of finite energy (that is, strong limits of sequences of smooth solutions in the topology induced by the energy, locally uniformly in time). Their existence and uniqueness for any finite-energy initial conditions was obtained in [⁠15⁠ J. Ginibre, A. Soffer and G. Velo, The global Cauchy problem for the critical nonlinear wave equation. J. Funct. Anal. 110, 96–130 (1992) , ⁠35⁠ J. Shatah and M. Struwe, Well-posedness in the energy space for semilinear wave equations with critical growth. Internat. Math. Res. Notices 1994, 303–309 (1994) ]. It can be deduced from Strichartz estimates for the wave equation, see for example [⁠5⁠ R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: I. Amer. J. Math. 137, 139–207 (2015) , Section 2] in the case $k \in {1, 2}$ . If $k \geq 3$ is large, Strichartz estimates from [⁠30⁠ F. Planchon, J. G. Stalker and A. S. Tahvildar-Zadeh, Lp estimates for the wave equation with the inverse-square potential. Discrete Contin. Dyn. Syst. 9, 427–442 (2003) ] can be applied, see [⁠19⁠ J. Jendrej and A. Lawrie, Two-bubble dynamics for threshold solutions to the wave maps equation. Invent. Math. 213, 1249–1325 (2018) , Section 2]. Finite-energy solutions of (⁠WM $_{k}$ ⁠) are not guaranteed to exist for all time. We denote $(T_{-}, T_{+}) \subset R$ the maximal time interval on which the solution exists.

3.2 Multibubble (multisoliton) configurations

Recalling the discussion from Section ⁠2.2⁠, the only $k$ -equivariant harmonic maps correspond to rational functions $a z^{k}, a z^{- k}, a \overline{z}^{k}$ and $a \overline{z}^{- k}$ , with $a > 0$ . In order to represent these maps in the context of the scalar equation (⁠WM $_{k}$ ⁠), it is convenient to denote

Q_{λ} (r) : = 2 arctan (\frac{r ^{k}}{λ ^{k}}), λ > 0.

Then the stationary solutions of (⁠WM $_{k}$ ⁠) are

Q_{λ} + 2 π ℓ, - Q_{λ} + 2 π ℓ, π + Q_{λ} + 2 π ℓ, π - Q_{λ} + 2 π ℓ,

for any $λ > 0$ and $ℓ \in Z$ . In this context of equation (⁠WM $_{k}$ ⁠), solitons are also called bubbles. Note that, with our notational conventions, $λ$ is the spatial scale of the bubble $Q_{λ}$ . For a given number of bubbles $M$ , an integer $m$ , and scales $0 < λ_{1} \leq λ_{2} \leq \dots \leq λ_{M}$ , we define a multibubble configuration by

Q (λ_{1}, \dots, λ_{M}) : = mπ + j = 1 \sum M \pm Q_{λ_{j}}

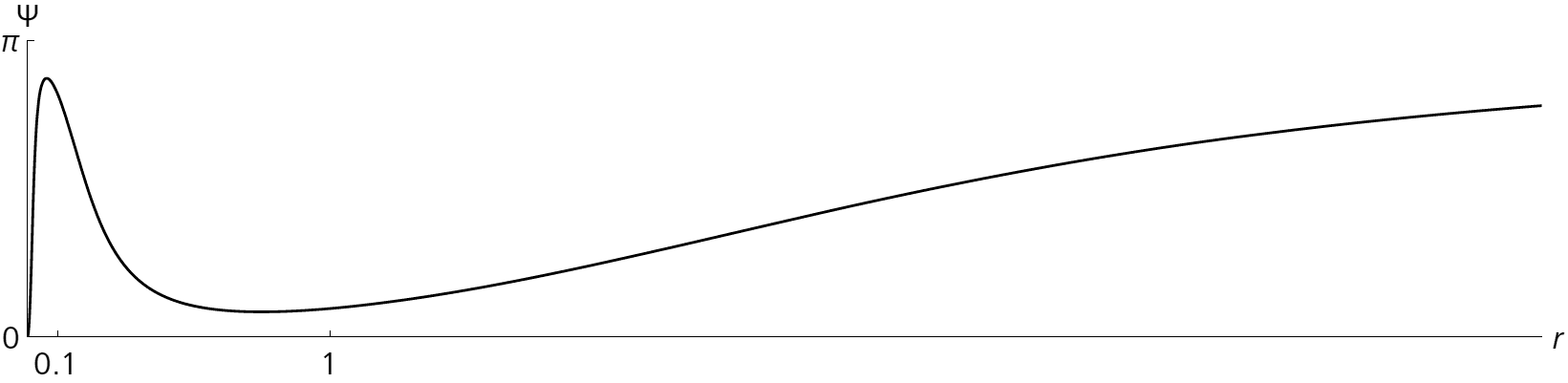
(in the notation, we skip the dependence on $m$ , which is not going to be essential here). One should think of the scales as satisfying $λ_{1} ≪ \dots ≪ λ_{M}$ , so that each bubble is separated in scale from all the others. Figure ⁠1⁠ shows a multibubble configuration with $(λ_{1}, λ_{2}, λ_{3}) = (\frac{1}{10}, \frac{1}{2}, 5)$ .

*Figure 1.* A multibubble configuration with three bubbles. 🅭🅯 CC BY 4.0

3.3 Soliton resolution

Our main result can be formulated as follows.

Theorem 3.2 (Jendrej–Lawrie []).

Let $ψ$ be a solution of (⁠WM $_{k}$ ⁠) defined for all $t \in (0, \infty)$ . As $t \to \infty$ , $(ψ (t, \cdot), \partial_{t} ψ (t, \cdot))$ decomposes into a superposition of

multibubble configuration,
radiation, corresponding to a solution of the linear wave equation (⁠W⁠),
remainder whose energy converges to $0$ .

Remark 3.3. We also prove a similar result in the case of a finite maximal time of existence of the solution.

Remark 3.4. The case $k = 1$ was settled by Duyckaerts, Kenig, Martel and Merle [⁠8⁠ T. Duyckaerts, C. Kenig, Y. Martel and F. Merle, Soliton resolution for critical co-rotational wave maps and radial cubic wave equation. Comm. Math. Phys. 391, 779–871 (2022) ] using a different approach (so-called channels of energy, see below).

Remark 3.5. It was proved in [⁠18⁠ J. Jendrej, Construction of two-bubble solutions for energy-critical wave equations. Amer. J. Math. 141, 55–118 (2019) ] that for all $k \geq 2$ , there exists a solution containing two bubbles. For these solutions, the radiation component vanishes. It was proved in [⁠21⁠ J. Jendrej and A. Lawrie, Soliton resolution for energy-critical wave maps in the equivariant case. J. Amer. Math. Soc. (electronically published, to appear in print) (2024) ] that for $k = 1$ multibubble solutions with vanishing radiation component do not exist. Existence of multibubble solutions with a non-vanishing radiation component is an open problem for all $k$ .

The history of the progress on understanding the dynamical behavior of large solutions of (⁠WM $_{k}$ ⁠) is quite long. Fundamental results were obtained in [⁠3⁠ D. Christodoulou and A. S. Tahvildar-Zadeh, On the regularity of spherically symmetric wave maps. Comm. Pure Appl. Math. 46, 1041–1091 (1993) , ⁠2⁠ D. Christodoulou and A. S. Tahvildar-Zadeh, On the asymptotic behavior of spherically symmetric wave maps. Duke Math. J. 71, 31–69 (1993) , ⁠37⁠ J. Shatah and A. Tahvildar-Zadeh, Regularity of harmonic maps from the Minkowski space into rotationally symmetric manifolds. Comm. Pure Appl. Math. 45, 947–971 (1992) , ⁠38⁠ J. Shatah and A. S. Tahvildar-Zadeh, On the Cauchy problem for equivariant wave maps. Comm. Pure Appl. Math. 47, 719–754 (1994) ], see also [⁠36⁠ J. Shatah and M. Struwe, Geometric wave equations. Courant Lect. Notes Math. 2, New York University, Courant Institute of Mathematical Sciences, New York; American Mathematical Society, Providence, RI (1998) , Chapter 8], the main conclusion being the decay of energy at the self-similar scale, which in particular excludes self-similar blow-up, but also, as proved by Struwe [⁠40⁠ M. Struwe, Equivariant wave maps in two space dimensions. Comm. Pure Appl. Math. 56, 815–823 (2003) ], leads to bubbling: if $ψ$ is a solution of (⁠WM $_{k}$ ⁠) which blows up in finite time $T_{+}$ , then there exist sequences $t_{n} \to T_{+}$ and $0 < λ_{n} ≪ T_{+} - t_{n}$ such that

(ψ (t_{n}, λ_{n} \cdot), λ_{n} \partial_{t} ψ (t_{n}, λ_{n} \cdot)) \to mπ \pm Q,

the convergence being understood in the topology induced by the energy locally (on bounded sets).

The bubbling also implies that a solution whose energy is smaller than the energy of $Q$ cannot blow up. It was proved by Côte, Kenig, Lawrie and Schlag [⁠5⁠ R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: I. Amer. J. Math. 137, 139–207 (2015) ] that such a solution actually has radiative behavior. Above this threshold energy, finite time blow-up can occur, as was proved in the works [⁠26⁠ J. Krieger, W. Schlag and D. Tataru, Renormalization and blow up for charge one equivariant critical wave maps. Invent. Math. 171, 543–615 (2008) , ⁠32⁠ I. Rodnianski and J. Sterbenz, On the formation of singularities in the critical O⁢(3)σ-model. Ann. of Math. (2) 172, 187–242 (2010) , ⁠31⁠ P. Raphaël and I. Rodnianski, Stable blow up dynamics for the critical co-rotational wave maps and equivariant Yang–Mills problems. Publ. Math. Inst. Hautes Études Sci. 115, 1–122 (2012) ] already mentioned above. Important progress toward the soliton resolution conjecture was made in [⁠6⁠ R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: II. Amer. J. Math. 137, 209–250 (2015) ]. Sequential soliton resolution, that is convergence to a superposition of solitons for a sequence of times, was proved by Côte [⁠4⁠ R. Côte, On the soliton resolution for equivariant wave maps to the sphere. Comm. Pure Appl. Math. 68, 1946–2004 (2015) ] for $k \in {1, 2}$ , and Jia and Kenig [⁠22⁠ H. Jia and C. Kenig, Asymptotic decomposition for semilinear wave and equivariant wave map equations. Amer. J. Math. 139, 1521–1603 (2017) ] for $k \geq 3$ . Similar results without imposing equivariant symmetry assumptions, but with a less precise description of the radiation, were obtained by Grinis [⁠16⁠ R. Grinis, Quantization of time-like energy for wave maps into spheres. Comm. Math. Phys. 352, 641–702 (2017) ].

In [⁠19⁠ J. Jendrej and A. Lawrie, Two-bubble dynamics for threshold solutions to the wave maps equation. Invent. Math. 213, 1249–1325 (2018) ], continuous in time resolution was proved at the minimal possible energy level allowing for existence of a two-bubble. As a relatively simple consequence, continuous in time resolution was proved in [⁠20⁠ J. Jendrej and A. Lawrie, Continuous time soliton resolution for two-bubble equivariant wave maps. Math. Res. Lett. 29, 1745–1766 (2022) ] under the assumption that the solution contains at most two bubbles.

For the closely related energy-critical wave equation, scattering below the ground state energy threshold was proved by Kenig and Merle [⁠24⁠ C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical focusing non-linear wave equation. Acta Math. 201, 147–212 (2008) ], establishing together with [⁠23⁠ C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical, focusing, non-linear Schrödinger equation in the radial case. Invent. Math. 166, 645–675 (2006) ] the so-called Kenig–Merle route map. In the radially symmetric case, the soliton resolution conjecture was proved by Duyckaerts, Kenig and Merle in space dimension $3$ in [⁠9⁠ T. Duyckaerts, C. Kenig and F. Merle, Classification of radial solutions of the focusing, energy-critical wave equation. Camb. J. Math. 1, 75–144 (2013) ], in any odd space dimension in [⁠10⁠ T. Duyckaerts, C. Kenig and F. Merle, Soliton resolution for the radial critical wave equation in all odd space dimensions. Acta Math. 230, 1–92 (2023) ], and in dimension $4$ in [⁠8⁠ T. Duyckaerts, C. Kenig, Y. Martel and F. Merle, Soliton resolution for critical co-rotational wave maps and radial cubic wave equation. Comm. Math. Phys. 391, 779–871 (2022) ] (in collaboration with Martel). All these works used the channels of energy introduced in [⁠9⁠ T. Duyckaerts, C. Kenig and F. Merle, Classification of radial solutions of the focusing, energy-critical wave equation. Camb. J. Math. 1, 75–144 (2013) ].

In the non-radial case, sequential soliton resolution was proved by Duyckaerts, Jia, Kenig and Merle [⁠7⁠ T. Duyckaerts, H. Jia, C. Kenig and F. Merle, Soliton resolution along a sequence of times for the focusing energy critical wave equation. Geom. Funct. Anal. 27, 798–862 (2017) ].

3.4 Main ideas of the proof

Let $ψ$ be a solution of (⁠WM $_{k}$ ⁠) defined for all $t \in (0, \infty)$ . Thanks to the sequential soliton resolution results [⁠4⁠ R. Côte, On the soliton resolution for equivariant wave maps to the sphere. Comm. Pure Appl. Math. 68, 1946–2004 (2015) , ⁠22⁠ H. Jia and C. Kenig, Asymptotic decomposition for semilinear wave and equivariant wave map equations. Amer. J. Math. 139, 1521–1603 (2017) ], we know that there exists a sequence $t_{n} \to \infty$ such that $(ψ (t_{n}, \cdot), \partial_{t} ψ (t_{n}, \cdot))$ decomposes into a superposition of a multibubble configuration, radiation and a small remainder. It thus suffices to prove a no-return lemma: if a multibubble configuration is destroyed (we say that a collision takes place), it cannot recover its shape (note the analogy with non-existence of homoclinic/heteroclinic orbits). A similar idea is present in the works of Duyckaerts and Merle [⁠11⁠ T. Duyckaerts and F. Merle, Dynamics of threshold solutions for energy-critical wave equation. Int. Math. Res. Pap. IMRP 2008, article no. rpn002 (2008) ], Nakanishi and Schlag [⁠29⁠ K. Nakanishi and W. Schlag, Global dynamics above the ground state energy for the focusing nonlinear Klein–Gordon equation. J. Differential Equations 250, 2299–2333 (2011) ], Krieger, Nakanishi and Schlag [⁠25⁠ J. Krieger, K. Nakanishi and W. Schlag, Global dynamics away from the ground state for the energy-critical nonlinear wave equation. Amer. J. Math. 135, 935–965 (2013) ] for a single soliton which is linearly unstable. In our case, interactions between solitons play a similar role as the linear instability in those works. This idea has already been used in [⁠19⁠ J. Jendrej and A. Lawrie, Two-bubble dynamics for threshold solutions to the wave maps equation. Invent. Math. 213, 1249–1325 (2018) ] in the special case where there are only two bubbles and the radiative component vanishes.

Funding. The author is supported by the ERC Starting Grant “INSOLIT” 101117126.

Jacek Jendrej is a professor at Sorbonne University. He studies dynamics of solutions of nonlinear wave equations, with a special emphasis on the role of solitons, multisolitons and dispersion. jendrej@imj-prg.fr

¹
This note is based on the talk given by the author at the 9th European Congress of Mathematics.

References

V. I. Arnol’d, Mathematical methods of classical mechanics. Second ed., Grad. Texts in Math. 60, Springer, New York (1989)
D. Christodoulou and A. S. Tahvildar-Zadeh, On the asymptotic behavior of spherically symmetric wave maps. Duke Math. J. 71, 31–69 (1993)
D. Christodoulou and A. S. Tahvildar-Zadeh, On the regularity of spherically symmetric wave maps. Comm. Pure Appl. Math. 46, 1041–1091 (1993)
R. Côte, On the soliton resolution for equivariant wave maps to the sphere. Comm. Pure Appl. Math. 68, 1946–2004 (2015)
R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: I. Amer. J. Math. 137, 139–207 (2015)
R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: II. Amer. J. Math. 137, 209–250 (2015)
T. Duyckaerts, H. Jia, C. Kenig and F. Merle, Soliton resolution along a sequence of times for the focusing energy critical wave equation. Geom. Funct. Anal. 27, 798–862 (2017)
T. Duyckaerts, C. Kenig, Y. Martel and F. Merle, Soliton resolution for critical co-rotational wave maps and radial cubic wave equation. Comm. Math. Phys. 391, 779–871 (2022)
T. Duyckaerts, C. Kenig and F. Merle, Classification of radial solutions of the focusing, energy-critical wave equation. Camb. J. Math. 1, 75–144 (2013)
T. Duyckaerts, C. Kenig and F. Merle, Soliton resolution for the radial critical wave equation in all odd space dimensions. Acta Math. 230, 1–92 (2023)
T. Duyckaerts and F. Merle, Dynamics of threshold solutions for energy-critical wave equation. Int. Math. Res. Pap. IMRP 2008, article no. rpn002 (2008)
W. Eckhaus and P. Schuur, The emergence of solitons of the Korteweg–de Vries equation from arbitrary initial conditions. Math. Methods Appl. Sci. 5, 97–116 (1983)
J. Eells and J. C. Wood, Restrictions on harmonic maps of surfaces. Topology 15, 263–266 (1976)
E. Fermi, J. Pasta and S. Ulam, Studies of nonlinear problems. Technical Report LA-1940, Los Alamos Scientific Laboratory, CA (1955)
J. Ginibre, A. Soffer and G. Velo, The global Cauchy problem for the critical nonlinear wave equation. J. Funct. Anal. 110, 96–130 (1992)
R. Grinis, Quantization of time-like energy for wave maps into spheres. Comm. Math. Phys. 352, 641–702 (2017)
F. Hélein, Harmonic maps, conservation laws and moving frames. Second ed., Cambridge Tracts in Math. 150, Cambridge University Press, Cambridge (2002)
J. Jendrej, Construction of two-bubble solutions for energy-critical wave equations. Amer. J. Math. 141, 55–118 (2019)
J. Jendrej and A. Lawrie, Two-bubble dynamics for threshold solutions to the wave maps equation. Invent. Math. 213, 1249–1325 (2018)
J. Jendrej and A. Lawrie, Continuous time soliton resolution for two-bubble equivariant wave maps. Math. Res. Lett. 29, 1745–1766 (2022)
J. Jendrej and A. Lawrie, Soliton resolution for energy-critical wave maps in the equivariant case. J. Amer. Math. Soc. (electronically published, to appear in print) (2024)
H. Jia and C. Kenig, Asymptotic decomposition for semilinear wave and equivariant wave map equations. Amer. J. Math. 139, 1521–1603 (2017)
C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical, focusing, non-linear Schrödinger equation in the radial case. Invent. Math. 166, 645–675 (2006)
C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical focusing non-linear wave equation. Acta Math. 201, 147–212 (2008)
J. Krieger, K. Nakanishi and W. Schlag, Global dynamics away from the ground state for the energy-critical nonlinear wave equation. Amer. J. Math. 135, 935–965 (2013)
J. Krieger, W. Schlag and D. Tataru, Renormalization and blow up for charge one equivariant critical wave maps. Invent. Math. 171, 543–615 (2008)
F. Linares and G. Ponce, Introduction to nonlinear dispersive equations. Second ed., Universitext, Springer, New York (2015)
N. Manton and P. Sutcliffe, Topological solitons. Cambridge Monogr. Math. Phys., Cambridge University Press, Cambridge (2004)
K. Nakanishi and W. Schlag, Global dynamics above the ground state energy for the focusing nonlinear Klein–Gordon equation. J. Differential Equations 250, 2299–2333 (2011)
F. Planchon, J. G. Stalker and A. S. Tahvildar-Zadeh, Lp estimates for the wave equation with the inverse-square potential. Discrete Contin. Dyn. Syst. 9, 427–442 (2003)
P. Raphaël and I. Rodnianski, Stable blow up dynamics for the critical co-rotational wave maps and equivariant Yang–Mills problems. Publ. Math. Inst. Hautes Études Sci. 115, 1–122 (2012)
I. Rodnianski and J. Sterbenz, On the formation of singularities in the critical O⁢(3)σ-model. Ann. of Math. (2) 172, 187–242 (2010)
J. Sacks and K. Uhlenbeck, The existence of minimal immersions of 2-spheres. Ann. of Math. (2) 113, 1–24 (1981)
H. Segur and M. J. Ablowitz, Asymptotic solutions and conservation laws for the nonlinear Schrödinger equation. I. J. Math. Phys. 17, 710–713 (1976)
J. Shatah and M. Struwe, Well-posedness in the energy space for semilinear wave equations with critical growth. Internat. Math. Res. Notices 1994, 303–309 (1994)
J. Shatah and M. Struwe, Geometric wave equations. Courant Lect. Notes Math. 2, New York University, Courant Institute of Mathematical Sciences, New York; American Mathematical Society, Providence, RI (1998)
J. Shatah and A. Tahvildar-Zadeh, Regularity of harmonic maps from the Minkowski space into rotationally symmetric manifolds. Comm. Pure Appl. Math. 45, 947–971 (1992)
J. Shatah and A. S. Tahvildar-Zadeh, On the Cauchy problem for equivariant wave maps. Comm. Pure Appl. Math. 47, 719–754 (1994)
M. Struwe, On the evolution of harmonic mappings of Riemannian surfaces. Comment. Math. Helv. 60, 558–581 (1985)
M. Struwe, Equivariant wave maps in two space dimensions. Comm. Pure Appl. Math. 56, 815–823 (2003)
T. Tao, Global regularity of wave maps. II. Small energy in two dimensions. Comm. Math. Phys. 224, 443–544 (2001)
T. Tao, Nonlinear dispersive equations: Local and global analysis. CBMS Reg. Conf. Ser. Math. 106, American Mathematical Society, Providence, RI (2006)
D. Tataru, On global existence and scattering for the wave maps equation. Amer. J. Math. 123, 37–77 (2001)
G. B. Whitham, Linear and nonlinear waves. Pure and Applied Mathematics, John Wiley & Sons, New York (1999)
N. J. Zabusky and M. D. Kruskal, Interaction of ”solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965)

Cite this article

Jacek Jendrej, Recent progress on the problem of soliton resolution. Eur. Math. Soc. Mag. 135 (2025), pp. 5–11

DOI 10.4171/MAG/237

🅭🅯

This open access article is published by EMS Press under a CC BY 4.0 license, with the exception of logos and branding of the European Mathematical Society and EMS Press, and where otherwise noted.

Back to Issue 135

[bib-bib1] V. I. Arnol’d, Mathematical methods of classical mechanics. Second ed., Grad. Texts in Math. 60, Springer, New York (1989)

[bib-bib2] D. Christodoulou and A. S. Tahvildar-Zadeh, On the asymptotic behavior of spherically symmetric wave maps. Duke Math. J. 71, 31–69 (1993)

[bib-bib3] D. Christodoulou and A. S. Tahvildar-Zadeh, On the regularity of spherically symmetric wave maps. Comm. Pure Appl. Math. 46, 1041–1091 (1993)

[bib-bib4] R. Côte, On the soliton resolution for equivariant wave maps to the sphere. Comm. Pure Appl. Math. 68, 1946–2004 (2015)

[bib-bib5] R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: I. Amer. J. Math. 137, 139–207 (2015)

[bib-bib6] R. Côte, C. E. Kenig, A. Lawrie and W. Schlag, Characterization of large energy solutions of the equivariant wave map problem: II. Amer. J. Math. 137, 209–250 (2015)

[bib-bib7] T. Duyckaerts, H. Jia, C. Kenig and F. Merle, Soliton resolution along a sequence of times for the focusing energy critical wave equation. Geom. Funct. Anal. 27, 798–862 (2017)

[bib-bib8] T. Duyckaerts, C. Kenig, Y. Martel and F. Merle, Soliton resolution for critical co-rotational wave maps and radial cubic wave equation. Comm. Math. Phys. 391, 779–871 (2022)

[bib-bib9] T. Duyckaerts, C. Kenig and F. Merle, Classification of radial solutions of the focusing, energy-critical wave equation. Camb. J. Math. 1, 75–144 (2013)

[bib-bib10] T. Duyckaerts, C. Kenig and F. Merle, Soliton resolution for the radial critical wave equation in all odd space dimensions. Acta Math. 230, 1–92 (2023)

[bib-bib11] T. Duyckaerts and F. Merle, Dynamics of threshold solutions for energy-critical wave equation. Int. Math. Res. Pap. IMRP 2008, article no. rpn002 (2008)

[bib-bib12] W. Eckhaus and P. Schuur, The emergence of solitons of the Korteweg–de Vries equation from arbitrary initial conditions. Math. Methods Appl. Sci. 5, 97–116 (1983)

[bib-bib13] J. Eells and J. C. Wood, Restrictions on harmonic maps of surfaces. Topology 15, 263–266 (1976)

[bib-bib14] E. Fermi, J. Pasta and S. Ulam, Studies of nonlinear problems. Technical Report LA-1940, Los Alamos Scientific Laboratory, CA (1955)

[bib-bib15] J. Ginibre, A. Soffer and G. Velo, The global Cauchy problem for the critical nonlinear wave equation. J. Funct. Anal. 110, 96–130 (1992)

[bib-bib16] R. Grinis, Quantization of time-like energy for wave maps into spheres. Comm. Math. Phys. 352, 641–702 (2017)

[bib-bib17] F. Hélein, Harmonic maps, conservation laws and moving frames. Second ed., Cambridge Tracts in Math. 150, Cambridge University Press, Cambridge (2002)

[bib-bib18] J. Jendrej, Construction of two-bubble solutions for energy-critical wave equations. Amer. J. Math. 141, 55–118 (2019)

[bib-bib19] J. Jendrej and A. Lawrie, Two-bubble dynamics for threshold solutions to the wave maps equation. Invent. Math. 213, 1249–1325 (2018)

[bib-bib20] J. Jendrej and A. Lawrie, Continuous time soliton resolution for two-bubble equivariant wave maps. Math. Res. Lett. 29, 1745–1766 (2022)

[bib-bib21] J. Jendrej and A. Lawrie, Soliton resolution for energy-critical wave maps in the equivariant case. J. Amer. Math. Soc. (electronically published, to appear in print) (2024)

[bib-bib22] H. Jia and C. Kenig, Asymptotic decomposition for semilinear wave and equivariant wave map equations. Amer. J. Math. 139, 1521–1603 (2017)

[bib-bib23] C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical, focusing, non-linear Schrödinger equation in the radial case. Invent. Math. 166, 645–675 (2006)

[bib-bib24] C. E. Kenig and F. Merle, Global well-posedness, scattering and blow-up for the energy-critical focusing non-linear wave equation. Acta Math. 201, 147–212 (2008)

[bib-bib25] J. Krieger, K. Nakanishi and W. Schlag, Global dynamics away from the ground state for the energy-critical nonlinear wave equation. Amer. J. Math. 135, 935–965 (2013)

[bib-bib26] J. Krieger, W. Schlag and D. Tataru, Renormalization and blow up for charge one equivariant critical wave maps. Invent. Math. 171, 543–615 (2008)

[bib-bib27] F. Linares and G. Ponce, Introduction to nonlinear dispersive equations. Second ed., Universitext, Springer, New York (2015)

[bib-bib28] N. Manton and P. Sutcliffe, Topological solitons. Cambridge Monogr. Math. Phys., Cambridge University Press, Cambridge (2004)

[bib-bib29] K. Nakanishi and W. Schlag, Global dynamics above the ground state energy for the focusing nonlinear Klein–Gordon equation. J. Differential Equations 250, 2299–2333 (2011)

[bib-bib30] F. Planchon, J. G. Stalker and A. S. Tahvildar-Zadeh, Lp estimates for the wave equation with the inverse-square potential. Discrete Contin. Dyn. Syst. 9, 427–442 (2003)

[bib-bib31] P. Raphaël and I. Rodnianski, Stable blow up dynamics for the critical co-rotational wave maps and equivariant Yang–Mills problems. Publ. Math. Inst. Hautes Études Sci. 115, 1–122 (2012)

[bib-bib32] I. Rodnianski and J. Sterbenz, On the formation of singularities in the critical O⁢(3)σ-model. Ann. of Math. (2) 172, 187–242 (2010)

[bib-bib33] J. Sacks and K. Uhlenbeck, The existence of minimal immersions of 2-spheres. Ann. of Math. (2) 113, 1–24 (1981)

[bib-bib34] H. Segur and M. J. Ablowitz, Asymptotic solutions and conservation laws for the nonlinear Schrödinger equation. I. J. Math. Phys. 17, 710–713 (1976)

[bib-bib35] J. Shatah and M. Struwe, Well-posedness in the energy space for semilinear wave equations with critical growth. Internat. Math. Res. Notices 1994, 303–309 (1994)

[bib-bib36] J. Shatah and M. Struwe, Geometric wave equations. Courant Lect. Notes Math. 2, New York University, Courant Institute of Mathematical Sciences, New York; American Mathematical Society, Providence, RI (1998)

[bib-bib37] J. Shatah and A. Tahvildar-Zadeh, Regularity of harmonic maps from the Minkowski space into rotationally symmetric manifolds. Comm. Pure Appl. Math. 45, 947–971 (1992)

[bib-bib38] J. Shatah and A. S. Tahvildar-Zadeh, On the Cauchy problem for equivariant wave maps. Comm. Pure Appl. Math. 47, 719–754 (1994)

[bib-bib39] M. Struwe, On the evolution of harmonic mappings of Riemannian surfaces. Comment. Math. Helv. 60, 558–581 (1985)

[bib-bib40] M. Struwe, Equivariant wave maps in two space dimensions. Comm. Pure Appl. Math. 56, 815–823 (2003)

[bib-bib41] T. Tao, Global regularity of wave maps. II. Small energy in two dimensions. Comm. Math. Phys. 224, 443–544 (2001)

[bib-bib42] T. Tao, Nonlinear dispersive equations: Local and global analysis. CBMS Reg. Conf. Ser. Math. 106, American Mathematical Society, Providence, RI (2006)

[bib-bib43] D. Tataru, On global existence and scattering for the wave maps equation. Amer. J. Math. 123, 37–77 (2001)

[bib-bib44] G. B. Whitham, Linear and nonlinear waves. Pure and Applied Mathematics, John Wiley & Sons, New York (1999)

[bib-bib45] N. J. Zabusky and M. D. Kruskal, Interaction of ”solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965)