Francis William Lawvere was one of the most influential figures in the late 20th century and up till now, because of his drive to unify and simplify mathematics, by sharpening the tools of category theory. The following is an attempt of describing some of the milestones and visions in this process.
1 Continuum physics
Lawvere was born in February 1937, as son of a farmer in Muncie, Indiana. He studied physics at the University of Indiana, and there soon felt the need for more useable and explicit foundations for the reasoning employed, in particular in continuum physics. He was in Indiana a student of Clifford Truesdell, the founder of the Springer journal “Archive for Rational Mechanics and Analysis,” who had a similar foundational agenda. L. saw already at this time the need for a category-theoretic approach. One first step was to achieve a “categorical dynamics” (some of which was materialized in the late 1960s). A crucial step was his category-theoretic formulation of the formation of function spaces, in terms of universal properties (adjoint functors): Cartesian closed categories.
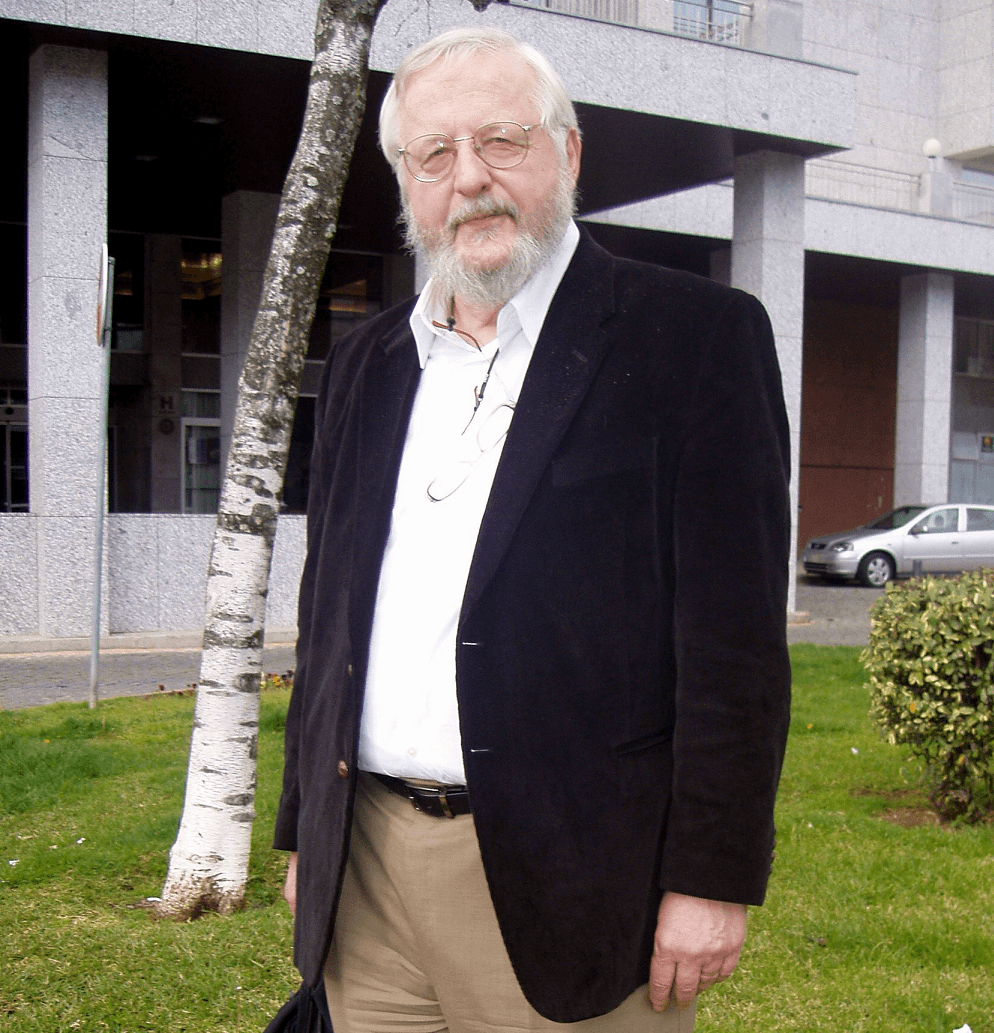
F. William Lawvere, Braga, March 2007 (© M. M. Clementino and J. Picado)
©All rights reserved.
Truesdell personally contacted Eilenberg to facilitate L.’s entrance into Columbia as Eilenberg’s Ph.D. student 1960–63 – with a break 1961–63, where L. went to California, to learn more set theory and logic from experts in the fields (Tarski, Scott and others). In the California period, L. finished his (Columbia) Ph.D. thesis on functorial semantics of algebraic theories, where in particular the notion of algebraic theory was given in a presentation-free way.
2 The Category of Sets
For L. himself, a turning point in his general search for useable and teachable foundations for mathematics was the year 1963–64 as an assistant professor at Reed College in Oregon. In an extensive interview with L., conducted in 2007 by Maria Manuel Clementino and Jorge Picado in Braga (Portugal) [2 M. M. Clemetino and J. Picado, Inteview with F. William Lawvere. http://www.mat.uc.pt/~picado/lawvere/interview.pdf (2007) ], L. says:
At Reed I was instructed that the first year of calculus should concentrate on foundations, formulas there being taught in the second year. Therefore […] I spent several preparatory weeks trying to devise a calculus course based on Zermelo–Fraenkel (ZF) set theory. However, a sober assessment showed that there are far too many layers of definitions, concealing differentiation and integration from the cumulative hierarchy, to be able to get through those layers in a year. The category structure of Cantor’s structureless sets seemed both simpler and closer. Thus, the elementary theory of the category of sets arose from a purely practical educational need.
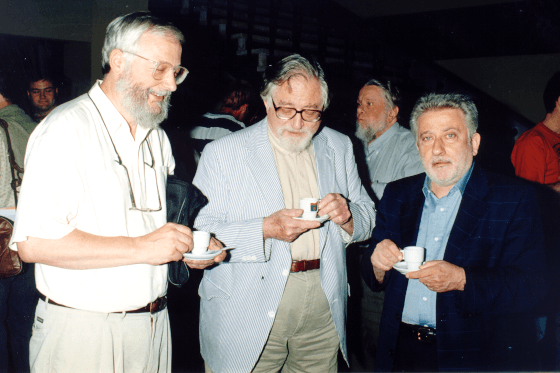
F. W. Lawvere, A. Heller, R. Lavendhomme (in the back) and A. Carboni at CT99, Coimbra, Portugal (© M. M. Clementino and J. Picado)
©All rights reserved.
Many of L.’s mathematical achievements (notions, constructions and theorems) result from efforts to improve the teaching of calculus and of engineering mathematics, and led him to conclude that a workable foundation for mathematics, even for a calculus course, cannot be formulated in terms of (membership), as in ZF, say, but can be formulated in terms of the notion of mappings (and their composition). L. says, in the Braga interview [2 M. M. Clemetino and J. Picado, Inteview with F. William Lawvere. http://www.mat.uc.pt/~picado/lawvere/interview.pdf (2007) ] 2007:
Philosophically, it may be said that these developments supported the thesis that even in set theory and elementary mathematics it was also true as has long been felt in advanced algebra and topology, namely that the substance of mathematics resides not in Substance, as it is made to seem when is the irreducible predicate, but in Form, as is clear when the guiding notion is isomorphism-invariant structure, as defined, for example, by universal mapping properties. As in algebra and topology, here again the concrete technical machinery for the precise expression and efficient handling of these ideas is provided by the Eilenberg–Mac Lane theory of categories, functors and natural transformations.
After the year at Reed College, L. went to Zürich, where he was visiting in 1964–66 at Beno Eckmann’s Forschungsinstitut für Mathematik. Eckmann had succeeded in attracting several category theorists to participate. Notably, the concept of monad (“triple”), and its relationships to algebraic theories and homology were elaborated (as documented in [3 B. Eckmann (ed.), Seminar on triples and categorical homology theory (ETH 1966/67). Lecture Notes in Math. 80, Springer, Berlin (1969) ]).
From Zürich, it was possible to attend seminars at the nearby Oberwolfach in South Germany. Here, L. met Peter Gabriel and learned from him aspects of Grothendieck’s approach to geometry, as expounded in SGA4 [1 M. Artin, A. Grothendieck and J. L. Verdier, Théorie des topos et cohomologie etale des schémas. Tome 1: Théorie des topos. Lecture Notes in Math. 269, Springer, Berlin (1972) ].
3 Grothendieck
Grothendieck’s work had a fundamental influence on L.’s later work. They first met each other at the ICM in Nice (1970), where they both were invited lecturers. L. had here publicly disagreed with Grothendieck in a separate lecture on his “Survival” movement.
In 1973, they were both visiting Buffalo. L. says in the Braga interview:
I vividly remember his tutoring me on basic insights of algebraic geometry, such as “points have automorphisms.” In 1981 I visited him in his stone hut, in the middle of a lavender field in the south of France, to ask his opinion of a project […]. My last meeting was at the same place in 1989 (Aurelio Carboni drove me there from Milano): he was clearly glad to see me but would not speak, the result of a religious vow; he wrote on paper that he was also forbidden to discuss mathematics, though quickly his mathematical soul triumphed, leaving me with some precious mathematical notes.
Lecturing in Coimbra, Portugal, March 1997 (© M. Sobral)
©All rights reserved.
4 Categorical dynamics and synthetic differential geometry
In most of 1967, L. was assistant professor at the University of Chicago. L. here began to apply Grothendieck’s topos theory in an advanced lecture series, centering around the problem of simplified foundations of continuum mechanics, inspired by Truesdell’s and Noll’s axiomatizations. The series was attended by Mac Lane, Jean Bénabou, Eduardo Dubuc and others, including the present author, who was at that time finishing a thesis, under L.’s supervision. The particular contribution which came out of the seminar was not yet a full-fledged categorical dynamics, but a kinematic basis for it: the idea of having the tangent bundle construction representable, for a postulated “infinitesimal” object (utilizing the Cartesian closed structure of the postulated category of spaces). An aspect of this “kinematic” line of thought was later developed by several people as a more full-fledged “synthetic differential geometry.”
The wisdom from algebraic geometry, which was at the basis of this development in categorical dynamics, could also be imported and applied in standard smooth differential geometry; L. uses an algebraic theory (in the sense of his 1963 thesis), namely the theory whose -ary operations are the smooth functions – a theory, for which it is crucial not to ask for a presentation in terms of generators and relations.
5 Elementary toposes, algebraic geometry and logic
L. returned to the Forschungsinstitut in Zürich in 1968–69. At this time, he had become more convinced that toposes were involved not only as a background for categorical dynamics, but also for notions from set theory and logic: boolean-valued models, and forcing (as in Cohen’s work (1963) on the continuum hypothesis). In the Braga interview, he says:
That these apparently totally different toposes, involving infinitesimal motion and advanced logic, could be part of the same simple axiomatic theory, was a promise in my 1967 Chicago course. It only became reality after my second stay at the Forschungsinstitut in Zürich, Switzerland 1968–69, during which I discovered the nature of the power set functor in toposes as a result of investigating the problem of expressing in elementary terms the operation of forming the associated sheaf, and after 1969–1970 […] through my collaboration with Myles Tierney.
This collaboration took place in Halifax (Canada): In 1969, L. had obtained the prestigious Killam professorship at Dalhousie University in Halifax, and was in that context allowed to invite a dozen collaborators (among them Tierney), likewise supported by Killam. This meant that during 1969–1971 Dalhousie became a lively place; mathematically, in particular, the notion of elementary topos gradually crystallized here. Significantly, L. had organized that a preprint version of (exposé I–IV) of SGA4 [1 M. Artin, A. Grothendieck and J. L. Verdier, Théorie des topos et cohomologie etale des schémas. Tome 1: Théorie des topos. Lecture Notes in Math. 269, Springer, Berlin (1972) ] was handed out to the participants of his seminar (SGA4 is Artin, Grothendieck and Verdier’s “Théorie des Topos et Cohomologie Etale des Schémas,” not officially published until 1972).
However, in 1971, the dream team at Dalhousie was dispersed; the university administration refused to renew the contract with L., due to his political activities in protesting against the Vietnam war and against the War Measures Act proclaimed by Trudeau, suspending civil liberties under the pretext of danger of terrorism. (But in 1995, Dalhousie hosted the celebration of 50 years of category theory, with participation of L.)
A conference organized by L., on the eve of his stay in Halifax in 1971, carries the significant title: “Toposes, Algebraic Geometry and Logic,” and the proceedings from this conference were published in 1972 [6 F. W. Lawvere (ed.), Toposes, algebraic geometry and logic. Lecture Notes in Math. 274, Springer, Berlin (1972) ].
After leaving Halifax in 1971, L. became visiting professor in Aarhus (Denmark) 1971–72, and in Perugia (Italy) 1972–73. These were years where the new insights in topos theory, brought about in Halifax, were consolidated and disseminated more widely. Also, after finally settling 1973 in Buffalo (US), L. maintained close contacts, in the form of shorter and longer stays, with his European friends and collaborators; this includes a year 1980–81 at IHÉS (Paris).
The toposes that we studied in Halifax and later, were in particular “gros toposes” (like the topos of simplicial sets), in contrast to the “petit toposes” (like the topos of sheaves on a topological space). This was a distinction made in SGA4, IV.4.10. This distinction was for L. one of the inputs of the study of the category of toposes, i.e., toposes in their functorial inter-relationship. Such studies were developed by many researchers, and documented in many mathematical monographs, articles, and in conferences (with or without proceedings). L. was very active in participating in conferences, often as invited keynote speaker; he was less active in getting the wealth of his ideas and visions down in written form. For instance, his seminal talks in Chicago in 1967 on categorical dynamics were not available in written form until in 1978, in the proceedings of a protracted “Open House” summer meeting in Aarhus, on “Topos Theoretic Methods in Geometry” [5 A. Kock (ed.), Topos theoretic methods in geometry, Various Publications Series 30, Aarhus University, Aarhus (1979) ].
In 1982, L. (together with his Buffalo colleague Steve Schanuel) organized a conference in Buffalo, “Categories in Continuum Physics,” with participation also of many key researchers in continuum physics, like Truesdell and Noll. Three of the articles in the proceedings (published in [8 F. W. Lawvere and S. H. Schanuel (eds.), Categories in continuum physics. Lecture Notes in Math. 1174, Springer, Berlin (1986) ]) deal with the problem of foundations of thermodynamics.
L. was in 1977 in the Scientific Steering Committee of the important and large summer meeting in Durham, “Applications of Sheaves” [4 M. P. Fourman, C. J. Mulvey and D. S. Scott (eds), Applications of sheaves. Proceedings of the research symposium on applications of sheaf theory to logic, algebra and analysis (Durham 1977), Lecture Notes in Math. 753, Springer, Berlin (1979) ], which marked a breakthrough in exploiting the relative simplicity of toposes in the conceptualization of mathematical and physical theories. L. gave a talk in Durham on “categories in the foundations of thermodynamics,” of which, however, I have not been able to find a written account. There does, on the other hand, exist accounts of a talk (with a lively debate) given by L. at this conference, with the title “The Logic of Mathematics,” where L. stated his view on the philosophy and development of mathematics. I include it here, since an obituary of L. would be incomplete, if it did not reflect the uncompromising character of his political/philosophical life and work:
In this Durham debate, L. says in the beginning of the talk (according to my notes and memory):
Mathematics is the science of space forms and quantitative relationships. What is the purpose of mathematics? Its purpose is to clarify this relationship in order to act as a basis of unity of people in solving problems (not mathematical problems) in the struggle for production, and in the conscientiousness of this struggle, which is scientific experimentation.
Already at this early stage of the talk came an interrupting question (possibly rhetoric) from a member of the audience: “What is the purpose of production?” L. thought for quite some time before answering: “To bring you here!”
Later on in the talk, L. stated:
The purpose of the logic of mathematics; to clarify and simplify the learning, use and development of mathematics. […] In a dialectical way: there is also a counterpurpose: to obscure, complicate and prevent the learning, use and development of mathematics. In particular, to freeze the development by promoting instead: thinking about forcing everything into a preconceived framework […]. Both of these purposes are fighting with each other inside each of us. […] Often the counterpurpose wins over the purpose. This is because the counterpurpose is in the interest of the ruling class. This is a thing which has changed drastically over the last 100 years. The interest of the monopoly capitalist class is against the development of production.
6 Axiomatic cohesion
This is not the place to give (nor would I be able to give) a complete survey of all the aspects of L.’s mathematical and philosophical work. Just some further key-words: probability, categorical logic, indexed/fibered categories, metric spaces as enriched categories, linguistics, extensive vs. intensive quantities, category of physical quantities, Grassmann, axiomatic cohesion.
The idea of axiomatic cohesion, as introduced by L. 2007 [7 F. W. Lawvere, Axiomatic cohesion. Theory Appl. Categ. 19, no. 3, 41–49 (2007) ], has in particular led to recent new developments.
The following is a quotation from this 2007 publication:
An explicit science of cohesion is needed to account for the varied background models for dynamical mathematical theories. Such a science needs to be sufficiently expressive to explain how these backgrounds are so different from other mathematical categories, and also different from one another, and yet so united that they can be mutually transformed. An everyday example of such mutual transformation is the weatherman’s application of the finite element method (which can be viewed as analysis in a combinatorial topos) to equations of continuum thermomechanics (which can be viewed as analysis in a smooth topos, where smooth functions and distributions live).
F. W. Lawvere with the author at Cafe Odeon, Zürich, Fall of 1966 (© A. Kock)
©All rights reserved.
The basis for this axiomatic science of cohesion is a string of four functors
each one in the string left adjoint to the next one. An example of such a string is familiar in topology: associating to a (sufficiently nice) space its set of connected components, associating to a set the discrete space structure on that set, associating to a space its set of points, and finally associating to a set the codiscrete space structure on that set. In the category of toposes, properties of such strings formulate many of the distinctions asked for in the above quotation.
Only some of the many the ideas that L. launched have reached written, let alone published, form, but exist only in the form of seeds in minds and notes of people who have been around.
Probably, many fruitful plants will emerge in the future from these seeds. The germination of the seeds would be enhanced if they were more accessible in some archive. Some activity in creating such archives is taking place, notably in www.acsu.buffalo.edu/~wlawvere.
References
- M. Artin, A. Grothendieck and J. L. Verdier, Théorie des topos et cohomologie etale des schémas. Tome 1: Théorie des topos. Lecture Notes in Math. 269, Springer, Berlin (1972)
- M. M. Clemetino and J. Picado, Inteview with F. William Lawvere. http://www.mat.uc.pt/~picado/lawvere/interview.pdf (2007)
- B. Eckmann (ed.), Seminar on triples and categorical homology theory (ETH 1966/67). Lecture Notes in Math. 80, Springer, Berlin (1969)
- M. P. Fourman, C. J. Mulvey and D. S. Scott (eds), Applications of sheaves. Proceedings of the research symposium on applications of sheaf theory to logic, algebra and analysis (Durham 1977), Lecture Notes in Math. 753, Springer, Berlin (1979)
- A. Kock (ed.), Topos theoretic methods in geometry, Various Publications Series 30, Aarhus University, Aarhus (1979)
- F. W. Lawvere (ed.), Toposes, algebraic geometry and logic. Lecture Notes in Math. 274, Springer, Berlin (1972)
- F. W. Lawvere, Axiomatic cohesion. Theory Appl. Categ. 19, no. 3, 41–49 (2007)
- F. W. Lawvere and S. H. Schanuel (eds.), Categories in continuum physics. Lecture Notes in Math. 1174, Springer, Berlin (1986)
- F. W. Lawvere and S. H. Schanuel, Conceptual mathematics. Cambridge University Press, Cambridge (1997) (2nd ed. 2009)
Cite this article
Anders Kock, F. William Lawvere (1937–2023): A lifelong struggle for the unity of mathematics. Eur. Math. Soc. Mag. 128 (2023), pp. 36–40
DOI 10.4171/MAG/143