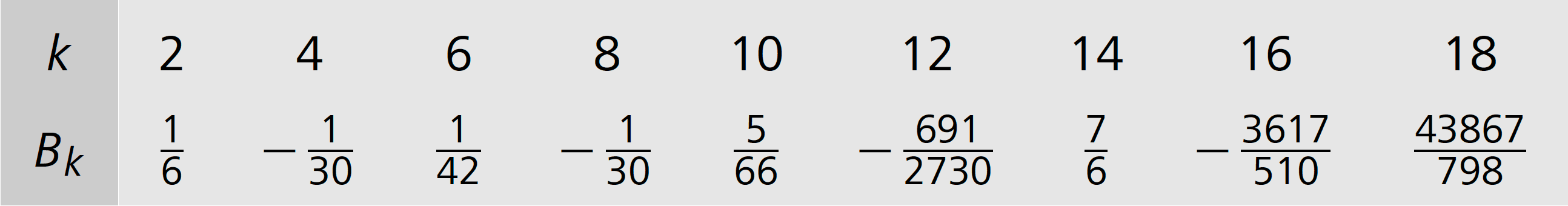Stable homotopy groups

1 Introduction

Homotopy theory studies homotopy invariants of topological spaces, i.e., invariants that are stable under continuous deformations. The fundamental problem is to understand the classification of continuous maps between spaces under homotopy.

In most situations, the spaces of interest are cellular, i.e., the spaces built from spheres in various dimensions. In this sense, spheres are the basic building blocks of spaces, and we would like to understand homotopy classes of maps from spheres to general spaces. By taking concatenation of maps, the homotopy classes of based maps from the $n$-sphere $S^{n}$ to a space $X$ form a group for $n\geq 1$, which is called the $n$-th homotopy group of $X$. When $n\geq 2$, there are different ways to concatenate maps and the resulting homotopy groups are commutative.

When $X$ is a simply connected finite CW complex, Serre [⁠41⁠ J.-P. Serre, Groupes d’homotopie et classes de groupes abéliens. Ann. of Math. (2) 58, 258–294 (1953) ] proved that all homotopy groups of $X$ are finitely generated abelian groups. So we can localize at a fixed prime $p$ when studying these groups, and once we understand the $p$-local parts for all $p$, the structures of the original groups can be recovered.

In this article, we give a survey of the stable part of the homotopy groups of spheres. We will first recall the notion of stable homotopy, and then discuss an interpretation in terms of the framed cobordism and an application to the classification of exotic spheres. In the last part we discuss some methods for computing these stable homotopy groups.

2 Stabilization of homotopy groups

One basic operation in homotopy theory is the suspension. For a pointed space $X$, its (reduced) suspension $\Sigma\,X$ is defined to be the smash product of $X$ with $S^{1}$, i.e., the quotient space $X\times S^{1}/X\vee S^{1}$. Roughly speaking, the effect of the suspension operation is to increase the dimension of all cells (other than the based point) of $X$ by one. For example, $\Sigma\,S^{n}\cong S^{n+1}$. The suspension operation is functorial, so we have a suspension homomorphism $\pi_{n}(X)\rightarrow\pi_{n+1}(\Sigma\,X)$. The celebrated Freudenthal suspension theorem says that it is an isomorphism when $X$ is sufficiently connected:

In particular, the groups $\pi_{n+k}(\Sigma^{n}X)$ depend only on $k$ when $n$ is sufficiently large, and we define this group to be the $k$-th stable homotopy group of $X$, denoted by $\pi_{k}^{s}(X)$. In contrast to the unstable homotopy groups, the stable homotopy groups form a generalized homology theory. This fact makes stable computations much simpler than those in the unstable cases.

The stabilization process can be categorified. We can define the (infinity) category of finite spectra by formally inverting the suspension functor on the category of finite CW complexes. The category of spectra is then defined as the ind-category of finite spectra. (See Lurie [⁠27⁠ J. Lurie, Derived algebraic geometry I: Stable ∞-categories. arXiv:math/0608228v5 (2009) , Section 9] for details.) From the definition it follows that, for any space $X$, there is an associated suspension spectrum $\Sigma^{\infty}X$. The stable homotopy group $\pi_{k}^{s}(X)$ is the group of homotopy classes of maps from $\Sigma^{\infty}S^{k}$ to $\Sigma^{\infty}X$ in the category of spectra.

The computation of the stable homotopy groups of the sphere spectrum $\Sigma^{\infty}S^{0}$ has a long history. It is easy to see that the group $\pi_{n}^{s}(S^{0})$ is trivial for $n<0$, and $\pi_{0}^{s}(S^{0})\cong\mathbb{Z}$ by the Hopf degree theorem. Using geometric methods, works of Hopf [⁠19⁠ H. Hopf, Über die Abbildungen der dreidimensionalen Sphäre auf die Kugelfläche. Math. Ann. 104, 637–665 (1931) ], Freudenthal [⁠16⁠ H. Freudenthal, Über die Klassen der Sphärenabbildungen I. Große Dimensionen. Compositio Math. 5, 299–314 (1938) ], Whitehead [⁠57⁠ G. W. Whitehead, The (n+2)nd homotopy group of the n-sphere. Ann. of Math. (2) 52, 245–247 (1950) ], Pontryagin [⁠36⁠ L. S. Pontryagin, Homotopy classification of the mappings of an (n+2)-dimensional sphere on an n-dimensional one. Doklady Akad. Nauk SSSR (N.S.) 70, 957–959 (1950) ] and Rokhlin [⁠39⁠ V. A. Rokhlin, The classification of mappings of the (n+3)-sphere to the n-sphere. Doklady Akad. Nauk SSSR (N.S.) 81, 19–22 (1951) ] determined $\pi_{n}^{s}(S^{0})$ for $n\leq 3$. Serre started the study of homotopy groups using algebraic machinery. In [⁠40⁠ J.-P. Serre, Homologie singulière des espaces fibrés. Applications. Ann. of Math. (2) 54, 425–505 (1951) ] Serre computed the homology of iterated loop spaces using the Serre spectral sequence and determined $\pi_{n}^{s}(S^{0})$ for $n<9$. Toda [⁠48⁠ H. Toda, Composition methods in homotopy groups of spheres. Ann. of Math. Stud. 49, Princeton University Press, Princeton (1962) ] introduced the method of secondary compositions, the Toda brackets. By studying the EHP sequence with the composition method, Toda determined $\pi_{n}^{s}(S^{0})$ for $n\leq 19$.

The introduction of the stable homotopy category by Spanier–Whitehead [⁠44⁠ E. H. Spanier and J. H. C. Whitehead, A first approximation to homotopy theory. Proc. Nat. Acad. Sci. U.S.A. 39, 655–660 (1953) ] and Boardman [⁠9⁠ M. Boardman, Stable homotopy theory. Mimeographed notes, The John Hopkins University (1965) ] brought to light the analogy between homotopy theory and homological algebra. Adams [⁠1⁠ J. F. Adams, On the structure and applications of the Steenrod algebra. Comment. Math. Helv. 32, 180–214 (1958) ] introduced the Adams spectral sequence, which can be thought of as the descent spectral sequence using the Eilenberg–MacLane spectrum as a cover for the sphere spectrum. Other covers, such as using the complex cobordism spectrum, give a more general Adams–Novikov spectral sequence. May [⁠29⁠ J. P. May, The cohomology of restricted Lie algebras and of Hopf algebras; application to the Steenrod algebra. Thesis, The Department of Mathematics, Princeton University (1964) ], Barratt–Mahowald–Tangora [⁠7⁠ M. G. Barratt, M. E. Mahowald and M. C. Tangora, Some differentials in the Adams spectral sequence. II. Topology 9, 309–316 (1970) ], Bruner [⁠12⁠ R. Bruner, A new differential in the Adams spectral sequence. Topology 23, 271–276 (1984) ], Nakamura [⁠34⁠ O. Nakamura, Some differentials in the mod⁡3 Adams spectral sequence. Bull. Sci. Engrg. Div. Univ. Ryukyus Math. Natur. Sci. 19, 1–25 (1975) ], Tangora [⁠47⁠ M. Tangora, Some homotopy groups mod 3. In Conference on homotopy theory (Evanston, 1974), Notas Mat. Simpos. 1, Soc. Mat. Mexicana, México, 227–245 (1975) ], Aubry [⁠4⁠ M. Aubry, Calculs de groupes d’homotopie stables de la sphère, par la suite spectrale d’Adams-Novikov. Math. Z. 185, 45–91 (1984) ] and Ravenel [⁠38⁠ D. C. Ravenel, Complex cobordism and stable homotopy groups of spheres. Pure Applied Math. 121, Academic Press, Orlando (1986) ] studied the Adams (and the Adams–Novikov) spectral sequence using techniques such as the May spectral sequence, the Massey product, Toda brackets, power operations, and the chromatic spectral sequence, etc., and determined $\pi_{n}^{s}(S^{0})$ up to $n=45$ at the prime $2$, up to $n=108$ for $p=3$, and up to $n=999$ for ${p=5}$. See also [⁠54⁠ G. Wang and Z. Xu, A survey of computations of homotopy groups of spheres and cobordisms. Preprint, https://sites.google.com/view/xuzhouli/research (2010) ] for a survey of classical methods. Recently, Isaksen [⁠21⁠ D. C. Isaksen, Stable stems. Mem. Amer. Math. Soc. 262, American Mathematical Society, Providence (2019) ] and Isaksen–Wang–Xu [⁠22⁠ D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres. Proc. Natl. Acad. Sci. USA 117, 24757–24763 (2020) , ⁠23⁠ D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres: From dimension 0 to 90. Publ. Math. Inst. Hautes Études Sci., 137, 107–243 (2023) ] made progress by using motivic methods, extending the knowledge of the $p=2$ component of $\pi_{n}^{s}(S^{0})$ up to $n=90$.

3 Framed cobordism

The Pontryagin–Thom construction gives a geometric interpretation of the homotopy groups of spheres.

Suppose we have a smooth map $f:S^{n+k}\rightarrow S^{n}$. Take a generic point $x_{0}\in S^{n}$. Then the pre-image $f^{-1}(x_{0})$ is a $k$-dimensional submanifold of $S^{n}$. Moreover, the normal bundle of $f^{-1}(x_{0})$ is the pull-back of the normal bundle of $x_{0}$ in $S^{n}$, so it is a trivial bundle and has a preferred trivialization. Pontryagin showed that $\pi_{n+k}(S^{n})$ is isomorphic to the group of cobordism classes consisting of $k$-dimensional submanifolds of $S^{n+k}$ equipped with a framing on the normal bundle.

The Pontryagin–Thom construction can be stabilized. The special case of the Freudenthal suspension theorem for spheres can be deduced from the Whitney embedding theorem. Once the background space is of sufficiently large dimension, the cobordism classification of $k$-manifolds becomes independent of the embedding. In particular, we have:

For simplicity, manifolds with framings of their stable normal bundles will be referred to as framed manifolds (to be distinguished from manifolds with framings on their tangent bundles).

Using Pontryagin’s theorem, one can see immediately that $\pi_{n}(S^{n})\cong\mathbb{Z}$ for $n\geq 1$. Using the knowledge of $\pi_{1}(SO(n))$ and the classification of $1$-manifolds, one can show that ${\pi_{3}(S^{2})\cong\mathbb{Z}}$ and $\pi_{n+1}(S^{n})\cong\mathbb{Z}/2$ for $n\geq 3$, generated by the Hopf map (i.e., the attaching map in $\mathbb{C}P^{2}$) and its suspensions.

The geometric computation of the second stable homotopy group of spheres is more subtle. One has to take care of the framings on the normal bundle of surfaces. Given such a surface and an essential loop on it, the obstruction to filling the loop and extending the framing is an element in $\pi_{1}(SO)\cong\mathbb{Z}/2$ (where $SO=\operatorname{colim}_{n}SO(n)$). It turns out this obstruction is quadratic in the mod $2$ homology class of the loop, and the obstruction for the framed surface to be a boundary is the Arf invariant of this quadratic form. It follows that $\pi_{2}^{s}(S^{0})\cong\mathbb{Z}/2$. See [⁠36⁠ L. S. Pontryagin, Homotopy classification of the mappings of an (n+2)-dimensional sphere on an n-dimensional one. Doklady Akad. Nauk SSSR (N.S.) 70, 957–959 (1950) ] for detailed arguments.

There is a special class of framed manifolds, consisting of those whose underlying manifolds are the standard spheres. Since all framings on the sphere $S^{k}$ can be classified by the group $\pi_{k}(SO)$, we have the $J$-homomorphism

introduced by Whitehead [⁠56⁠ G. W. Whitehead, On the homotopy groups of spheres and rotation groups. Ann. of Math. (2) 43, 634–640 (1942) ]. The image of $J$ was computed by Adams [⁠3⁠ J. F. Adams, On the groups J⁢(X). IV. Topology 5, 21–71 (1966) ] in terms of the Adams conjecture, which was later proved by Quillen [⁠37⁠ D. Quillen, The Adams conjecture. Topology 10, 67–80 (1971) ] and Sullivan [⁠46⁠ D. Sullivan, Genetics of homotopy theory and the Adams conjecture. Ann. of Math. (2) 100, 1–79 (1974) ]:

Recall that the Bernoulli number is defined by the generating function

By the von Staudt–Clausen theorem, it follows that the order of $\pi_{k}^{s}(S^{0})$ is unbounded as $k$ increases. The following is a list of some Bernoulli numbers:

4 Exotic spheres

The classification of manifolds with the homotopy type of the sphere is a long-standing problem in topology, starting with Poincaré’s famous conjecture on simply connected $3$-manifolds. By works of Smale [⁠42⁠ S. Smale, Generalized Poincaré’s conjecture in dimensions greater than four. Ann. of Math. (2) 74, 391–406 (1961) ], Freedman [⁠15⁠ M. H. Freedman, The topology of four-dimensional manifolds. J. Differential Geometry 17, 357–453 (1982) ] and Perelman [⁠35⁠ G. Perelman, Finite extinction time for the solutions to the Ricci flow on certain three-manifolds. arXiv:math/0307245 (2003) ], all homotopy spheres are homeomorphic to the standard sphere. For the smooth classification, in dimension $2$ and $3$, any manifold has a unique smooth structure, according to work by Moise [⁠31⁠ E. E. Moise, Affine structures in 3-manifolds. V. The triangulation theorem and Hauptvermutung. Ann. of Math. (2) 56, 96–114 (1952) ]. In dimension $4$, it is still unknown if there exist exotic $4$-spheres. In dimension $\geq 5$, we can classify exotic spheres by Kervaire–Milnor theory in terms of stable homotopy groups of spheres.

For $n\geq 5$, we let $\Theta_{n}$ be the set of smooth structures on the spheres. (By the h-cobordism theorem of Smale [⁠43⁠ S. Smale, On the structure of manifolds. Amer. J. Math. 84, 387–399 (1962) ], this is the same as the classification of h-cobordism classes of homotopy spheres.) It forms an abelian group under connected sum. Kervaire–Milnor [⁠26⁠ M. A. Kervaire and J. W. Milnor, Groups of homotopy spheres. I. Ann. of Math. (2) 77, 504–537 (1963) ] introduced a two-step strategy to study $\Theta_{n}$. First we classify the homotopy spheres up to framed cobordism, and then classify the homotopy spheres that bound framed manifolds.

One can prove that all homotopy spheres admit stable framings, and the choices of the different framings are cosets by the image of $J$. So we get a homomorphism

One needs to understand the kernel and the cokernel of this map. We let $\Theta_{n}^{bp}$ denote the kernel, which consists of the homotopy spheres that bound framed manifolds.

The study of the cokernel amounts to the following:

This question can be studied with the surgery theory, introduced by Milnor [⁠30⁠ J. Milnor, A procedure for killing homotopy groups of differentiable manifolds. In Proc. Sympos. Pure Math., Vol. III, American Mathematical Society, Providence, 39–55 (1961) ]. Suppose $X$ is an $n$-manifold. A surgery on $X$ is to first remove from $X$ an embedded $D^{k}\times S^{n-k}$, and then to fill its boundary $S^{k-1}\times S^{n-k}$ along the other direction with $S^{k-1}\times D^{n-k+1}$. When $X$ is framed, one needs to pay additional care to extend the framing. The operation of surgery is exactly what happens to the level set of a Morse function when crossing a critical point. So preforming surgery does not change the cobordism class and in fact generates the equivalence relation of cobordism.

For a framed $n$-manifold, one can perform suitable surgeries to kill all homotopy groups below the middle dimension. By Poincaré duality, in odd dimensions we would end up with a homotopy sphere. For $n$ even, the intersection form in the middle-dimensional cohomology enters the scene. If $n=4k$, then the obstruction to killing the middle cohomology is the signature of the intersection form. Since our manifold has trivial stable normal bundle, by Hirzebruch’s signature theorem, this obstruction vanishes and we end with a homotopy sphere. In the case when $n=4k+2$, similar to the situation in dimension $2$, we can define a quadratic form on the modulo $2$ cohomology, and the obstruction to getting a homotopy sphere via surgery is its Arf invariant. This is called the Kervaire invariant, originally introduced by Kervaire [⁠25⁠ M. A. Kervaire, A manifold which does not admit any differentiable structure. Comment. Math. Helv. 34, 257–270 (1960) ] to construct topological manifolds that admit no smooth structures. In summary, a framed cobordism class of dimension $n=4k+2$ contains a homotopy sphere if and only if its Kervaire invariant is trivial.

To understand the structure of $\Theta_{n}^{bp}$, we start with a homotopy sphere which bounds a framed $(n+1)$-manifold $X$. Then again we try to do surgery on $X$ to make it contractible. If this can be achieved, then by the h-cobordism theorem, the boundary will be the standard sphere when $n\geq 5$. As before we can kill homotopy classes below the middle dimension, and for $n+1$ odd there are no obstructions, so $\Theta_{n}^{bp}=0$. When $n+1=4k$, the obstruction to killing the middle dimension is the signature of the intersection form, which can be any multiple of $8$ using the plumbing construction. There is another operation we can perform, namely, taking the connected sum with a framed manifold whose boundary is a standard sphere. The boundaries of these objects are classified by the kernel of the $J$-homomorphism. Using Theorem ⁠3⁠ and Hirzebruch’s signature theorem, the effect of this operation is fully understood. Finally, if $n+1=4k+2$, then the obstruction for the middle-dimensional surgery is the Kervaire invariant, which can take any value in $\mathbb{Z}/2$. Again we can alter $X$ by taking the connected sum with a closed framed manifold, so this obstruction either becomes trivial, or does not depend on the existence of a closed framed $(n+1)$-manifold of Kervaire invariant $1$. In summary:

Together with the knowledge of the stable homotopy groups of spheres, we can partially answer the question: In which dimensions does the sphere have a unique smooth structure? Based on Serre’s computations [⁠40⁠ J.-P. Serre, Homologie singulière des espaces fibrés. Applications. Ann. of Math. (2) 54, 425–505 (1951) ] and Toda’s computations [⁠48⁠ H. Toda, Composition methods in homotopy groups of spheres. Ann. of Math. Stud. 49, Princeton University Press, Princeton (1962) ], Kervaire and Milnor found that $S^{5}$, $S^{6}$, $S^{12}$ have a unique smooth structure. Isaksen’s computation [⁠21⁠ D. C. Isaksen, Stable stems. Mem. Amer. Math. Soc. 262, American Mathematical Society, Providence (2019) ] implies that $S^{56}$ also has a unique smooth structure. The last sphere we know of that has a unique smooth structure is $S^{61}$, by work of Wang–Xu [⁠55⁠ G. Wang and Z. Xu, The triviality of the 61-stem in the stable homotopy groups of spheres. Ann. of Math. (2) 186, 501–580 (2017) ]. This solves the problem in all odd dimensions.

In even dimensions, by Behrens–Hill–Hopkins–Mahowald [⁠8⁠ M. Behrens, M. Hill, M. J. Hopkins and M. Mahowald, Detecting exotic spheres in low dimensions using coker⁡J. J. Lond. Math. Soc. (2) 101, 1173–1218 (2020) ], the only spheres below dimension $140$ which have unique smooth structures are $S^{2}$, $S^{6}$, $S^{12}$, $S^{56}$ and perhaps $S^{4}$. Based on the above results, we have following conjecture:

5 The Adams spectral sequence

A basic homotopy invariant is cohomology. Maps inducing non-trivial homomorphisms on cohomology are not homotopic to constant maps. To get finer invariants, we consider cohomology operations.

Cohomology operations are natural transformations of cohomology theories. To understand stable homotopy, we usually consider stable cohomology operations, i.e., the ones commuting with the suspension. The Bockstein homomorphism is such a stable operation. More generally, the Steenrod reduced power operation (see Steenrod–Epstein [⁠45⁠ N. E. Steenrod, Cohomology operations. Ann. of Math. Stud. 50, Princeton University Press, Princeton (1962) ]), which arises from the Spanier–Whitehead dual of the diagonal map, turns out to be stable. Since (ordinary) cohomology theories are represented by the Eilenberg–MacLane spectra, the stable cohomology operations can be classified by the cohomology of these objects, which was computed by Cartan [⁠13⁠ H. Cartan, Sur les groupes d’Eilenberg–Mac Lane. II, Proc. Nat. Acad. Sci. U.S.A. 40, 704–707 (1954) ].

The algebra $\mathcal{A}^{*}$ is called the Steenrod algebra.

One can use these cohomology operations to detect non-trivial maps that induce trivial homomorphisms in cohomology. For example, consider the Hopf map $\eta:S^{3}\rightarrow S^{2}$ and its mapping cone, i.e., the complex projective plane $\mathbb{C}P^{2}$. The Steenrod square $Sq^{2}$ acts non-trivially on the mod 2 cohomology of $\mathbb{C}P^{2}$, and consequently $\eta$ represents a non-trivial stable class in $\pi_{1}^{s}(S^{0})$.

In general, if there is a map $f:X\rightarrow Y$ that induces the trivial map on mod $p$ cohomology, then we have a short exact sequence

Here $Cf$ is the mapping cone of $f$ and we abbreviate $H^{*}(\,\cdot\,;\mathbb{F}_{p})$ by $H^{*}(\,\cdot\,)$. These cohomology operations act on every term, so this is a short exact sequence of $\mathcal{A}^{*}$-modules, and therefore it corresponds to an element in $\operatorname{Ext}^{1}_{\mathcal{A}^{*}}(H^{*}(Y),H^{*-1}(X))$.

More generally, suppose a map $f:X\rightarrow Y$ can be written as the composition of a sequence of maps

such that each $f_{i}$ induces a trivial map on mod $p$ cohomology. Then we have an element in $\operatorname{Ext}^{1}_{\mathcal{A}^{*}}(H^{*}(X_{i+1}),H^{*-1}(X_{i}))$ for each $i$, and composing them together gives an element in $\operatorname{Ext}^{n}_{\mathcal{A}^{*}}(H^{*}(Y),{}H^{*-n}(X))$. In contrast to the $n=1$ case, the decomposition of $f$ is not necessarily unique, and in general different decompositions yield different classes in the group $\operatorname{Ext}^{n}_{\mathcal{A}^{*}}(H^{*}(Y),H^{*-n}(X))$. However, we will see below that we do get an invariant by taking the cosets by certain subgroups of $\operatorname{Ext}^{n}_{\mathcal{A}^{*}}(H^{*}(Y),H^{*-n}(X))$ (which are hit by some Adams differentials).

The method of the Adams spectral sequence introduced by Adams [⁠1⁠ J. F. Adams, On the structure and applications of the Steenrod algebra. Comment. Math. Helv. 32, 180–214 (1958) ] is in some sense taking the universal example of the above decomposition. For a spectrum $Y$, an Adams tower is a sequence of maps $\dots\rightarrow Y_{2}\rightarrow Y_{1}\rightarrow Y$ such that each map induces a trivial homomorphism in mod $p$ cohomology, with its cofiber being homotopy equivalent to a wedge sum of (suspensions of) Eilenberg–MacLane spectra. The spectral sequence associated to an Adams tower is called the Adams spectral sequence. Adams towers always exist, and different towers for the same $Y$ always induce the same spectral sequence from the $E_{2}$-page. Moreover, the Adams $E_{2}$-page is the Ext groups over the Steenrod algebra.

For general spectra that are not necessarily finite, we still have the Adams spectral sequence, but the convergence issue is more subtle. See Bousfield [⁠10⁠ A. K. Bousfield, The localization of spectra with respect to homology. Topology 18, 257–281 (1979) ] for details.

We say an element $f\in[\Sigma^{*}X,Y]$ has Adams filtration $\geq n$ if it factors through $Y_{n}$ in an Adams tower. Then this Adams tower gives a decomposition of $f$ in the form (⁠1⁠). The corresponding element in $\operatorname{Ext}^{n}_{\mathcal{A}^{*}}(H^{*}(Y),H^{*}(X))$ is the element detecting $f$ in the $E_{\infty}$-page of the Adams spectral sequence. (Here we adopt the convention that $0\in\operatorname{Ext}^{n}_{\mathcal{A}^{*}}(H^{*}(Y),H^{*}(X))$ “detects” elements with Adams filtration $\geq n+1$.)

If $X$ and $Y$ are both the sphere spectrum, then the composition induces a ring structure on $\pi_{*}^{s}(S^{0})$, which is commutative (in the graded sense). In this case, the Adams spectral sequence is multiplicative. The multiplication on the $E_{2}$-page is the Yoneda product on Ext groups, which also turns out to be commutative (in the graded sense).

Let us give some examples of elements in $\pi_{*}^{s}(S^{0})$ with low Adams filtrations.

The identity map is essentially the only class with Adams filtration $0$. For Adams filtration $1$, note that $\operatorname{Ext}^{1}_{\mathcal{A}^{*}}(\mathbb{F}_{p},\mathbb{F}_{p})$ is generated by the indecomposable elements in $\mathcal{A}^{*}$, which turns out to be the vector space with basis $\{Sq^{2^{i}}\text{ for }i=0,1,\dots\}$ at the prime $2$. We denote by $h_{i}$ the class that corresponds to $Sq^{2^{i}}$.

The multiplication by $2$ map is detected by $h_{0}$ in the Adams $E_{2}$-page. The previous example regarding the Hopf map tells us that $\eta$ is detected by $h_{1}$. Furthermore, the attaching maps in projective planes over the quaternions and octonions give us elements $\nu\in\pi_{3}^{s}(S^{0})$ and $\sigma\in\pi_{7}^{s}(S^{0})$ that are detected by $h_{2}$ and $h_{3}$, respectively. We cannot produce more examples along this way because there are no more division algebras over the real numbers. In fact, Adams [⁠2⁠ J. F. Adams, On the non-existence of elements of Hopf invariant one. Ann. of Math. (2) 72, 20–104 (1960) ] proved that all the $h_{i}$’s for $i\geq 4$ do not survive in the Adams spectral sequence, and consequently there are no more Hopf invariant one classes. As a consequence, $S^{1}$, $S^{3}$, $S^{7}$ are the only spheres that have a trivial tangent bundle.

By computations of Adams [⁠2⁠ J. F. Adams, On the non-existence of elements of Hopf invariant one. Ann. of Math. (2) 72, 20–104 (1960) ], $\operatorname{Ext}^{2}_{\mathcal{A}^{*}}(\mathbb{F}_{2},\mathbb{F}_{2})$ is spanned by elements of the form $h_{i}h_{j}$ under the relations $h_{i}h_{i+1}=0$. Among these classes are the Kervaire classes $h_{i}^{2}$. By Browder’s theorem [⁠11⁠ W. Browder, The Kervaire invariant of framed manifolds and its generalization. Ann. of Math. (2) 90, 157–186 (1969) ], the Kervaire invariant for framed manifolds is trivial in dimensions other than $2^{n}-2$, and the existence of framed $(2^{n}-2)$-manifold with Kervaire invariant one is equivalent to the statement that $h_{n-1}^{2}$ survives in the Adams spectral sequence.

By the existence of $\eta$, $\nu$ and $\sigma$, we deduce that there exist Kervaire-invariant one manifolds in dimensions $2$, $6$ and $14$. In fact, one can take manifolds $S^{1}\times S^{1}$, $S^{3}\times S^{3}$ and ${S^{7}\times S^{7}}$ with suitable framings. Mahowald–Tangora [⁠28⁠ M. Mahowald and M. Tangora, Some differentials in the Adams spectral sequence. Topology 6, 349–369 (1967) ] and Barratt–Jones–Mahowald [⁠6⁠ M. G. Barratt, J. D. S. Jones and M. E. Mahowald, Relations amongst Toda brackets and the Kervaire invariant in dimension 62. J. London Math. Soc. (2) 30, 533–550 (1984) ] proved (see also Xu [⁠58⁠ Z. Xu, The strong Kervaire invariant problem in dimension 62. Geom. Topol. 20, 1611–1624 (2016) ]) that the elements $h_{4}^{2}$ and $h_{5}^{2}$ survive in the Adams spectral sequence. Using equivariant methods, Hill–Hopkins–Ravenel [⁠18⁠ M. A. Hill, M. J. Hopkins and D. C. Ravenel, On the nonexistence of elements of Kervaire invariant one. Ann. of Math. (2) 184, 1–262 (2016) ] proved that for ${n\geq 7}$, $h_{n}^{2}$ all support non-trivial differentials, and consequently the last dimension where there could exist a Kervaire-invariant one manifold is 126.

6 Motivic homotopy theory

In general, it is hard to determine differentials and hidden extensions in the Adams spectral sequence. Various techniques are used, but none of them can solve all the problems. This phenomenon is described as the Mahowald uncertainty principle; see [⁠24⁠ D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres and motivic homotopy theory. In Proceedings of the International Congress of Mathematicians (2022), DOI 10.4171/ICM2022/32 (to appear) ] for more details. Nevertheless, one of the most recent technique involves motivic homotopy theory and it turns out to be very effective.

The original motivation for developing motivic homotopy theory in Morel [⁠32⁠ F. Morel, Théorie homotopique des schémas. Astérisque 256, Société Mathématique de France, Paris (1999) ] and Morel–Voevodsky [⁠33⁠ F. Morel and V. Voevodsky, 𝐀1-homotopy theory of schemes. Inst. Hautes Études Sci. Publ. Math. 90, 45–143 (1999) ] is to construct a homotopy theory in the world of algebraic varieties. From the perspective of topologists whose main focus is classical homotopy theory, motivic homotopy theory is obtained by adding new objects in the world of topological spaces.

In classical homotopy theory, simplices are the basic building blocks. The classical homotopy category is equivalent to the category of simplicial sets, i.e., presheaves over simplices. In general, a category of presheaves can be viewed as the category freely generated from certain building blocks. The motivic category is constructed by first formally adding smooth varieties along with simplices as basic building blocks. In contrast to simplices, smooth varieties are not “independent”, in the sense that two varieties can be glued together to form a new one. To incorporate these relations, we consider simplicial sheaves (under certain Grothendieck topology, the most fruitful one being the Nisnevich topology) over the category of smooth varieties, instead of just presheaves. Finally, we invert $\mathbb{A}^{1}$-homotopy equivalences to get the motivic homotopy category. See Morel–Voevodsky [⁠33⁠ F. Morel and V. Voevodsky, 𝐀1-homotopy theory of schemes. Inst. Hautes Études Sci. Publ. Math. 90, 45–143 (1999) ] for details of this construction.

An interesting fact in the motivic world is that there are two kinds of spheres, the simplicial sphere $S^{1,0}$ and the multiplicative group $S^{1,1}=\mathbb{G}_{m}$ (the sheaf represented by the punctured affine line). Taking the smash product of these objects, we obtain motivic spheres $S^{i,j}$, where the first index $i$ indicates the dimension and the second index $j$ is the motivic weight. They are analogs of representation spheres in equivariant homotopy theory. To construct the stable motivic homotopy category, we mimic the construction in the equivariant setting, inverting suspensions with respect to both kinds of spheres. Analogously, we can define the notion of stable motivic homotopy groups, and as a result there are two gradings.

Now suppose we work with the base field $\mathbb{C}$. These two kinds of spheres are related by an element $\tau$ constructed as follows. At a prime $p$, for any $n$, we take a $p^{n}$-th root of unity, which induces a map $S^{0,0}\rightarrow S^{1,1}$, representing an element in $\pi_{0,0}^{s}(S^{1,1})$ of order $p^{n}$. So it is the image of some element $\tau_{n}\in\pi_{1,0}^{s}(S^{1,1};\mathbb{Z}/p^{n})$ under the Bockstein homomorphism. When we take a compatible system of $p^{n}$-th roots of unity for all $n$, then the resulting $\tau_{n}$’s are compatible, and we define $\tau$ to be the limit of $\tau_{n}$ in $\pi_{1,0}^{s}(S^{1,1};\mathbb{Z}_{p})$, which can be viewed as a self-map of the $p$-completed sphere of degree $(0,-1)$. Intuitively, $\tau$ can be regarded as the Bockstein pre-image of the infinitesimal generator of the multiplicative group. See Hu–Kriz–Ormsby [⁠20⁠ P. Hu, I. Kriz and K. Ormsby, Remarks on motivic homotopy theory over algebraically closed fields. J. K-Theory 7, 55–89 (2011) ] for more details.

By works of Voevodsky [⁠52⁠ V. Voevodsky, The Milnor conjecture. Preprint (2010) , ⁠49⁠ V. Voevodsky, Motivic cohomology with 𝐙/2-coefficients. Publ. Math. Inst. Hautes Études Sci. 59–104 (2003) , ⁠50⁠ V. Voevodsky, Reduced power operations in motivic cohomology. Publ. Math. Inst. Hautes Études Sci. 1–57 (2003) , ⁠51⁠ V. Voevodsky, Motivic Eilenberg–MacLane spaces. Publ. Math. Inst. Hautes Études Sci. 1–99 (2010) ], as in the classical case, we can define motivic cohomology, motivic Steenrod algebra and motivic Adams spectral sequence. Over $\mathbb{C}$, the coefficient ring of mod $p$ motivic cohomology is a polynomial ring $\mathbb{F}_{p}[\tau]$ generated by $\tau$. The motivic Steenrod algebra $\mathcal{A}_{\mathrm{mot}}^{*,*}$ is generated by a motivic analog of the Steenrod reduced powers, satisfying a motivic analog of the Adem relations.

There is a Betti realization functor from the motivic homotopy category to the classical homotopy category, induced by the functor sending a complex analytic variety over $\mathbb{C}$ to its underlying topological space. Under the Betti realization, the map $\tau$ becomes an equivalence and the two kinds of motivic spheres become classically equivalent. Moreover, Dugger–Isaksen [⁠14⁠ D. Dugger and D. C. Isaksen, The motivic Adams spectral sequence. Geom. Topol. 14, 967–1014 (2010) ] proved that the $\tau$-inverted motivic Adams spectral sequence for the motivic sphere recovers the classical Adams spectral sequence for the classical sphere. So intuitively we find that (after $p$-completion) the classical homotopy theory is the $\tau$-inverted motivic homotopy theory.

From a computational perspective, we can view $\tau$ as a deformation parameter of the motivic deformation. The generic fiber is the world of classical homotopy theory. Gheorghe–Wang–Xu [⁠17⁠ B. Gheorghe, G. Wang and Z. Xu, The special fiber of the motivic deformation of the stable homotopy category is algebraic. Acta Math. 226, 319–407 (2021) ] discovered that the special fiber lands in the algebraic world:

The latter algebraic category can be further identified with the derived category of quasi-coherent sheaves over the moduli stack of $p$-completed formal groups.

In particular, the Adams spectral sequence in the category of $S^{0,0}/\tau$-modules is also algebraic in nature. In fact, we have the following:

Recall that the algebraic Novikov spectral sequence computes the Ext groups of $BP_{*}BP$-comodules using the filtration by powers of the augmentation ideal of $BP_{*}$. The structure of the algebraic Novikov spectral sequence can be determined effectively with a computer using a minimal resolution. See Wang [⁠53⁠ G. Wang, Computations of the Adams–Novikov E2-term. Chinese Ann. Math. Ser. B 42, 551–560 (2021) ] for an algorithm of this computation.

So in principle we can get information on the special fiber of the motivic deformation as far as we wish. To get information on the classical homotopy theory, we try to propagate the information from the special fiber to the generic fiber of this motivic deformation. In practice, we use the $\tau$-Bockstein spectral sequence. We have a square of four spectral sequences:

One notes that the algebraic $\tau$-Bockstein spectral sequence is equivalent to the motivic analog of the classical Cartan–Eilenberg spectral sequence, and the $\tau$-Bockstein spectral sequence is equivalent to the motivic analog of the classical Adams–Novikov spectral sequence. Hence, our theorem links these classical objects through motivic theory and we are able to compare data obtained from different classical perspectives.

As an illustration of the method, we compute the first few Adams differentials in stem $15$. By Theorem ⁠11⁠ and computer output, there is a motivic Adams differential $d_{2}(h_{4})=h_{0}h_{3}^{2}$ for $S^{0,0}/\tau$. By comparison using the map $S^{0,0}\rightarrow S^{0,0}/\tau$, we find that in the motivic Adams spectral sequence for $S^{0,0}$, $h_{4}$ must support a non-zero differential of length at most $2$. The only possibility is that $d_{2}(h_{4})=h_{0}h_{3}^{2}$ also holds for $S^{0,0}$. By inverting $\tau$, we arrive at the same differential for the classical Adams spectral sequence for the sphere. The differential $d_{3}(h_{0}h_{4})=h_{0}d_{0}$ can be proved similarly. In fact, all non-zero differentials up to stem $45$ can be computed in this way, with very few exceptions. See the appendix of Gheorghe–Wang–Xu [⁠17⁠ B. Gheorghe, G. Wang and Z. Xu, The special fiber of the motivic deformation of the stable homotopy category is algebraic. Acta Math. 226, 319–407 (2021) ] for more details.

Acknowledgements. The first author acknowledges the support of NSFC-12226002, Shanghai Rising-Star Program 20QA1401600, and Shanghai Pilot Program for Basic Research-FuDan University 21TQ1400100(21TQ002). The second author acknowledges the support of the NSF under grant DMS 2105462.

Guozhen Wang is a professor of mathematics at Shanghai Center for Mathematical Sciences, Fudan University. wangguozhen@fudan.edu.cn Zhouli Xu is an associate professor of mathematics at the University of California, San Diego. xuzhouli@ucsd.edu

References

1. J. F. Adams, On the structure and applications of the Steenrod algebra. Comment. Math. Helv. 32, 180–214 (1958)
2. J. F. Adams, On the non-existence of elements of Hopf invariant one. Ann. of Math. (2) 72, 20–104 (1960)
3. J. F. Adams, On the groups J⁢(X). IV. Topology 5, 21–71 (1966)
4. M. Aubry, Calculs de groupes d’homotopie stables de la sphère, par la suite spectrale d’Adams-Novikov. Math. Z. 185, 45–91 (1984)
5. T. Bachmann, H. J. Kong, G. Wang and Z. Xu, The Chow t-structure on the ∞-category of motivic spectra. Ann. of Math. (2) 195, 707–773 (2022)
6. M. G. Barratt, J. D. S. Jones and M. E. Mahowald, Relations amongst Toda brackets and the Kervaire invariant in dimension 62. J. London Math. Soc. (2) 30, 533–550 (1984)
7. M. G. Barratt, M. E. Mahowald and M. C. Tangora, Some differentials in the Adams spectral sequence. II. Topology 9, 309–316 (1970)
8. M. Behrens, M. Hill, M. J. Hopkins and M. Mahowald, Detecting exotic spheres in low dimensions using coker⁡J. J. Lond. Math. Soc. (2) 101, 1173–1218 (2020)
9. M. Boardman, Stable homotopy theory. Mimeographed notes, The John Hopkins University (1965)
10. A. K. Bousfield, The localization of spectra with respect to homology. Topology 18, 257–281 (1979)
11. W. Browder, The Kervaire invariant of framed manifolds and its generalization. Ann. of Math. (2) 90, 157–186 (1969)
12. R. Bruner, A new differential in the Adams spectral sequence. Topology 23, 271–276 (1984)
13. H. Cartan, Sur les groupes d’Eilenberg–Mac Lane. II, Proc. Nat. Acad. Sci. U.S.A. 40, 704–707 (1954)
14. D. Dugger and D. C. Isaksen, The motivic Adams spectral sequence. Geom. Topol. 14, 967–1014 (2010)
15. M. H. Freedman, The topology of four-dimensional manifolds. J. Differential Geometry 17, 357–453 (1982)
16. H. Freudenthal, Über die Klassen der Sphärenabbildungen I. Große Dimensionen. Compositio Math. 5, 299–314 (1938)
17. B. Gheorghe, G. Wang and Z. Xu, The special fiber of the motivic deformation of the stable homotopy category is algebraic. Acta Math. 226, 319–407 (2021)
18. M. A. Hill, M. J. Hopkins and D. C. Ravenel, On the nonexistence of elements of Kervaire invariant one. Ann. of Math. (2) 184, 1–262 (2016)
19. H. Hopf, Über die Abbildungen der dreidimensionalen Sphäre auf die Kugelfläche. Math. Ann. 104, 637–665 (1931)
20. P. Hu, I. Kriz and K. Ormsby, Remarks on motivic homotopy theory over algebraically closed fields. J. K-Theory 7, 55–89 (2011)
21. D. C. Isaksen, Stable stems. Mem. Amer. Math. Soc. 262, American Mathematical Society, Providence (2019)
22. D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres. Proc. Natl. Acad. Sci. USA 117, 24757–24763 (2020)
23. D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres: From dimension 0 to 90. Publ. Math. Inst. Hautes Études Sci., 137, 107–243 (2023)
24. D. C. Isaksen, G. Wang and Z. Xu, Stable homotopy groups of spheres and motivic homotopy theory. In Proceedings of the International Congress of Mathematicians (2022), DOI 10.4171/ICM2022/32 (to appear)
25. M. A. Kervaire, A manifold which does not admit any differentiable structure. Comment. Math. Helv. 34, 257–270 (1960)
26. M. A. Kervaire and J. W. Milnor, Groups of homotopy spheres. I. Ann. of Math. (2) 77, 504–537 (1963)
27. J. Lurie, Derived algebraic geometry I: Stable ∞-categories. arXiv:math/0608228v5 (2009)
28. M. Mahowald and M. Tangora, Some differentials in the Adams spectral sequence. Topology 6, 349–369 (1967)
29. J. P. May, The cohomology of restricted Lie algebras and of Hopf algebras; application to the Steenrod algebra. Thesis, The Department of Mathematics, Princeton University (1964)
30. J. Milnor, A procedure for killing homotopy groups of differentiable manifolds. In Proc. Sympos. Pure Math., Vol. III, American Mathematical Society, Providence, 39–55 (1961)
31. E. E. Moise, Affine structures in 3-manifolds. V. The triangulation theorem and Hauptvermutung. Ann. of Math. (2) 56, 96–114 (1952)
32. F. Morel, Théorie homotopique des schémas. Astérisque 256, Société Mathématique de France, Paris (1999)
33. F. Morel and V. Voevodsky, 𝐀1-homotopy theory of schemes. Inst. Hautes Études Sci. Publ. Math. 90, 45–143 (1999)
34. O. Nakamura, Some differentials in the mod⁡3 Adams spectral sequence. Bull. Sci. Engrg. Div. Univ. Ryukyus Math. Natur. Sci. 19, 1–25 (1975)
35. G. Perelman, Finite extinction time for the solutions to the Ricci flow on certain three-manifolds. arXiv:math/0307245 (2003)
36. L. S. Pontryagin, Homotopy classification of the mappings of an (n+2)-dimensional sphere on an n-dimensional one. Doklady Akad. Nauk SSSR (N.S.) 70, 957–959 (1950)
37. D. Quillen, The Adams conjecture. Topology 10, 67–80 (1971)
38. D. C. Ravenel, Complex cobordism and stable homotopy groups of spheres. Pure Applied Math. 121, Academic Press, Orlando (1986)
39. V. A. Rokhlin, The classification of mappings of the (n+3)-sphere to the n-sphere. Doklady Akad. Nauk SSSR (N.S.) 81, 19–22 (1951)
40. J.-P. Serre, Homologie singulière des espaces fibrés. Applications. Ann. of Math. (2) 54, 425–505 (1951)
41. J.-P. Serre, Groupes d’homotopie et classes de groupes abéliens. Ann. of Math. (2) 58, 258–294 (1953)
42. S. Smale, Generalized Poincaré’s conjecture in dimensions greater than four. Ann. of Math. (2) 74, 391–406 (1961)
43. S. Smale, On the structure of manifolds. Amer. J. Math. 84, 387–399 (1962)
44. E. H. Spanier and J. H. C. Whitehead, A first approximation to homotopy theory. Proc. Nat. Acad. Sci. U.S.A. 39, 655–660 (1953)
45. N. E. Steenrod, Cohomology operations. Ann. of Math. Stud. 50, Princeton University Press, Princeton (1962)
46. D. Sullivan, Genetics of homotopy theory and the Adams conjecture. Ann. of Math. (2) 100, 1–79 (1974)
47. M. Tangora, Some homotopy groups mod 3. In Conference on homotopy theory (Evanston, 1974), Notas Mat. Simpos. 1, Soc. Mat. Mexicana, México, 227–245 (1975)
48. H. Toda, Composition methods in homotopy groups of spheres. Ann. of Math. Stud. 49, Princeton University Press, Princeton (1962)
49. V. Voevodsky, Motivic cohomology with 𝐙/2-coefficients. Publ. Math. Inst. Hautes Études Sci. 59–104 (2003)
50. V. Voevodsky, Reduced power operations in motivic cohomology. Publ. Math. Inst. Hautes Études Sci. 1–57 (2003)
51. V. Voevodsky, Motivic Eilenberg–MacLane spaces. Publ. Math. Inst. Hautes Études Sci. 1–99 (2010)
52. V. Voevodsky, The Milnor conjecture. Preprint (2010)
53. G. Wang, Computations of the Adams–Novikov E2-term. Chinese Ann. Math. Ser. B 42, 551–560 (2021)
54. G. Wang and Z. Xu, A survey of computations of homotopy groups of spheres and cobordisms. Preprint, https://sites.google.com/view/xuzhouli/research (2010)
55. G. Wang and Z. Xu, The triviality of the 61-stem in the stable homotopy groups of spheres. Ann. of Math. (2) 186, 501–580 (2017)
56. G. W. Whitehead, On the homotopy groups of spheres and rotation groups. Ann. of Math. (2) 43, 634–640 (1942)
57. G. W. Whitehead, The (n+2)nd homotopy group of the n-sphere. Ann. of Math. (2) 52, 245–247 (1950)
58. Z. Xu, The strong Kervaire invariant problem in dimension 62. Geom. Topol. 20, 1611–1624 (2016)