1 Introduction
This paper investigates a Diophantine equation derived from a principle of construction for musical harmonies and scales advocated in antiquity by Claudius Ptolemy (c. 85–160 AD), known also for his geocentric model of celestial motion. Ptolemy’s “Harmonics” was one of the main sources for Greek music theory in the Middle Ages and remained influential in the Renaissance up to the 17th century [3 A. Barker, Scientific method in Ptolemy’s “Harmonics”. Cambridge University Press, New Haven (2000) , 4 A. Barker, Mathematical beauty made audible: Musical aesthetics in Ptolemy’s “Harmonics”. Classical Philology 105, 403–420 (2010) , 16 J. Solomon, Ptolemy, “Harmonics”: Translation and commentary. Brill, Leiden (2000) ].
Retrospectively, the theory of proportions in the Pythagorean tradition can be considered a theory for rational numbers greater than one under multiplication1We are aware that the antique ratios are not yet fully fledged rational numbers, i.e., classes of ordered pairs of integers. Whereas ratios were considered equivalent to their representations in lowest terms, the order of the terms of a ratio was not constitutive. The ratios and , for instance, represent the same relationship (the epitriti or sesquitertia) corresponding to the musical interval of the fourth (the diatessaron, see Figure 1) in the sense of a perceptual distance. For our purpose the restriction to rational numbers greater than 1 is sufficient and convenient.. In its application to musical harmonies and scales, adding musical intervals corresponds to the multiplication of rational numbers, and therefore piling equal intervals, i.e., multiplying a given interval by an integer, is equivalent with raising its ratio to the respective integer power2The term musical interval refers to logarithms of frequency ratios. Many quantifiable sensory phenomena and their physical counterparts are in a logarithmic or nearly logarithmic relationship, as loudness or brightness sensation with respect to the intensity of sound or light..
All rights reserved.
According to Ptolemy’s music-aesthetic premises, the multiple and the so-called epimoric ratios are the building blocks of musical harmonies and scales. Epimoric ratios (also called superparticular ratios) are positive rational numbers of the form , whereas multiple ratios of the form are ordinary natural numbers. Since they can be written as the unit plus a unit fraction (), epimoric ratios can be regarded as an elementary form of improper fractions accessible to perception guided by the intellect, see [3 A. Barker, Scientific method in Ptolemy’s “Harmonics”. Cambridge University Press, New Haven (2000) , pp. 60–62], [4 A. Barker, Mathematical beauty made audible: Musical aesthetics in Ptolemy’s “Harmonics”. Classical Philology 105, 403–420 (2010) ] and [7 P. Gozza, Number to sound: The musical way to the scientific revolution. The Western Ontario Series in Philosophy of Science 64, Springer, Dordrecht (2000) , pp. 191–200]. The role of epimoric ratios in music theory and pitch perception was repeatedly emphasized and problematized throughout the course of history – in the second half of the 16th century for instance, they were debated by Gioseffo Zarlino (1517–1590) and Vincenzo Galilei3The father of Galileo. (1520–1591) [14 B. V. Rivera, Theory ruled by practice: Zarlino’s reversal of the classical system of proportions. Indiana Theory Review 16, 145–160 (1995) , 15 F. Sargolini, La critica di Vincenzo Galilei al misticismo numerico di Gioseffo Zarlino. Nuncius 15, DOI 10.1163/182539100X00038 (2000) ]. On the other hand, epimoric ratios also played a crucial role in the development of novel calculation techniques: Simon Stevin (1548/49–1620) used various epimoric bases in his tables of compound interest, and Jost Bürgi (1552–1632) created a fine-grained and very accurate exponential table with more than 23,000 entries for the epimoric base , see [17 S. Stevin, Tafelen van interest, midtgaders de constructie der selver … Christoffel Plantijn, Antwerpen (1582) ], [18 S. Stevin, The principal works of Simon Stevin. Vol. IIA: Mathematics. C. V. Swets & Zeitlinger, Amsterdam (1958) , p. 75] and [11 D. Muzzulini, The geometry of musical logarithms. Acta Musicologica 87, 193–216, DOI 10.5281/zenodo.5541790 (2015) , pp. 199–200, 209–210].
All rights reserved.
Archytas of Tarentum (c. 420–c. 350 BC) proved that the equation
has no integer solutions in for integer exponents (see [2 A. Barker, Ptolemy’s Pythagoreans, Archytas, and Plato’s conception of mathematics. Phronesis 39, 113–135 (1994) ]). Archytas’ reasoning was discussed by Boethius (c. 477–524 AD) [9 C. A. Huffman, Archytas of Tarentum: Pythagorean, philosopher and mathematician king. Cambridge University Press, Cambridge (2007) , pp. 451–470], who also quotes a more general result and its proof from the Euclidean “Sectio Canonis”4See [1 A. Barker, Greek musical writings: II Harmonic and acoustic theory. Cambridge University Press, Cambridge (1989) , p. 195]; the assignment of the “Sectio Canonis” to Euclid is insecure., stating that epimoric ratios can be decomposed in no way into products of two or more equal integer ratios – [5 Boethius, De institutione arithmetica libri duo: De institutione musica libri quinque. B. G. Teubner, Leipzig (1867) , Inst. Mus. IV.2, p. 303], [6 Boethius, Fundamentals of music. Yale University Press, New Haven (1989) , p. 118].
All rights reserved.
In other words, musical intervals of an epimoric ratio, such as the octave or the fourth , cannot be divided equally into smaller intervals of epimoric ratios, i.e., equal division results in “irrational ratios”5Irrationality had a precarious ontological status as being defined only ex negativo, and it was linked to incommensurable (geometric) quantities, see also [12 P. Pesic, Hearing the irrational: Music and the development of the modern concept of number. Isis 101, 501–530 (2010) ].. This fact makes it impossible to construct musical scales or scale segments with several equal intervals of epimoric ratios spanning for example a fourth or an octave. Our theorem, the main result of this paper, proves that a similar restriction holds for the partition of an epimoric ratio into a power of an epimoric ratio and a single epimoric cofactor: It shows that for positive integers and exponent , equation (1) below has no solutions6The conjecture that (1) has no solutions was formulated by the second author, from the study of music theory and on the basis of his mathematical background. It was eventually settled in a joint effort of the two authors.. Introducing an epimoric cofactor, , into the decomposition raises the upper bound for solvability only by .
2 The main theorem
We will prove the following theorem.
The Diophantine equation
has no integer solutions.
The following derivation holds for all . The value allows for an infinity of solutions; it will be considered in detail in a separate paper. By removing denominators, we obtain the following two equivalent formulations of (1):
The last identity in (3) implies that , so we can in particular divide by .
We define and note that
In both equations (2) and (3), we encounter various pairs of factors of the type for some , for instance in (2). These are coprime and in order to exploit this useful fact, we define a series of factors whose existence follows from such relations of coprimality. Namely, we set and . We assume and let the cofactors be and for some . Since , it follows that and . By combining the last relations of divisibility with the definitions of and , we get
Using the upper bound on in (4), we obtain the following estimates for .
Proof. The upper bound for follows from (5); the lower bound follows from the same identity, in conjunction with the upper bound in (4). Since , we obtain our first lower bound for , namely , hence . ∎
Our next task is to derive from the above and some additional bounds, a tight interval which must contain ; en route we also obtain sharper lower bounds on .
Lemma 2. Under the same assumption as above, we let
Then
Moreover, and there is at most one integer . In particular, if (1) has a solution, then .
Proof. We have
Now, and , so the previous becomes
Since , it follows that , and in (5), we find
Since , we get
hence . Reinserted in (5) with the definition , this leads to
So
thus
consequently,
Since , we conclude that
Statement (7) follows from these inequalities, by inserting the definitions of and . The length of the interval is ; the improved lower bound on will show that for , and thus the interval contains at most one integer, which confirms the statement on .
Now , so
Since , we also have . This will lead to the bound for . Assume first that . Then . But for , so we obtain a contradiction to , and thus , which is absurd.
It remains to treat the case . We decompose , so that all primes that divide are either coprime to , or occur in with a power less than , while . Then and (6) implies a fortiori that holds along with . Since , we have
and thus and a fortiori . In particular, must be large, namely
as claimed. Using this bound, a straightforward verification shows that , and this completes the proof of Lemma 2. ∎
We finally use the bound (10) and sharper estimates for to complete the proof of Theorem 1. If , then there are no solutions, and we are done. Otherwise, we let be the unique integer in the interval . Since is also an integer, it follows that .
Proof of Theorem 1. We determine in terms of . We have
with
Consequently,
where
Since , there is a number of the form , with and denoting the fractional part of , such that
We claim that . The definition of implies that
Since is an integer and , it follows that and , as claimed.
Thus, . Inserting this value of in (8) yields
hence
with
We have seen that , so if the left-hand side of (11) does not vanish, then its cofactor is an integer or a half-integer; if it does not vanish, its absolute value will exceed . We denoted the right-hand side of (11) by , so
For the right-hand side, small values of allow for larger values of , so we first assume . In this case
Now and inserting this in the bound for , we see that . Since the left-hand side is at least in absolute value, if it does not vanish, we conclude that the two sides must vanish simultaneously. Thus, and the right-hand side is . Since and for , the last expression cannot vanish for , so there are no solutions in this case.
The case is more delicate; recall that in this case and thus . The best bound for the error term is now , so in (11)
We generate a contradiction by a case-by-case examination. We know that , so . The cases and are easily seen to be impossible. In the first case, the right-hand side is too small, while in the second case it is too large, compared to , as one verifies from the definitions.
If , we obtain
thus . We have seen above that , while implies , and thus . But then , contradicting the upper bound established above. We conclude that there are no solutions for either, and this completes the proof. ∎
3 Remarks and comments
Here we provide some historical details that place our result in its musical context. For additional reading we recommend the excellent modern introduction to superparticular ratios7This is an other expression for epimoric ratios. by Halsey and Hewitt [8 G. D. Halsey and E. Hewitt, More on the superparticular ratios in music. Amer. Math. Monthly 79, 1096–1100 (1972) ].
3.1 Music theory
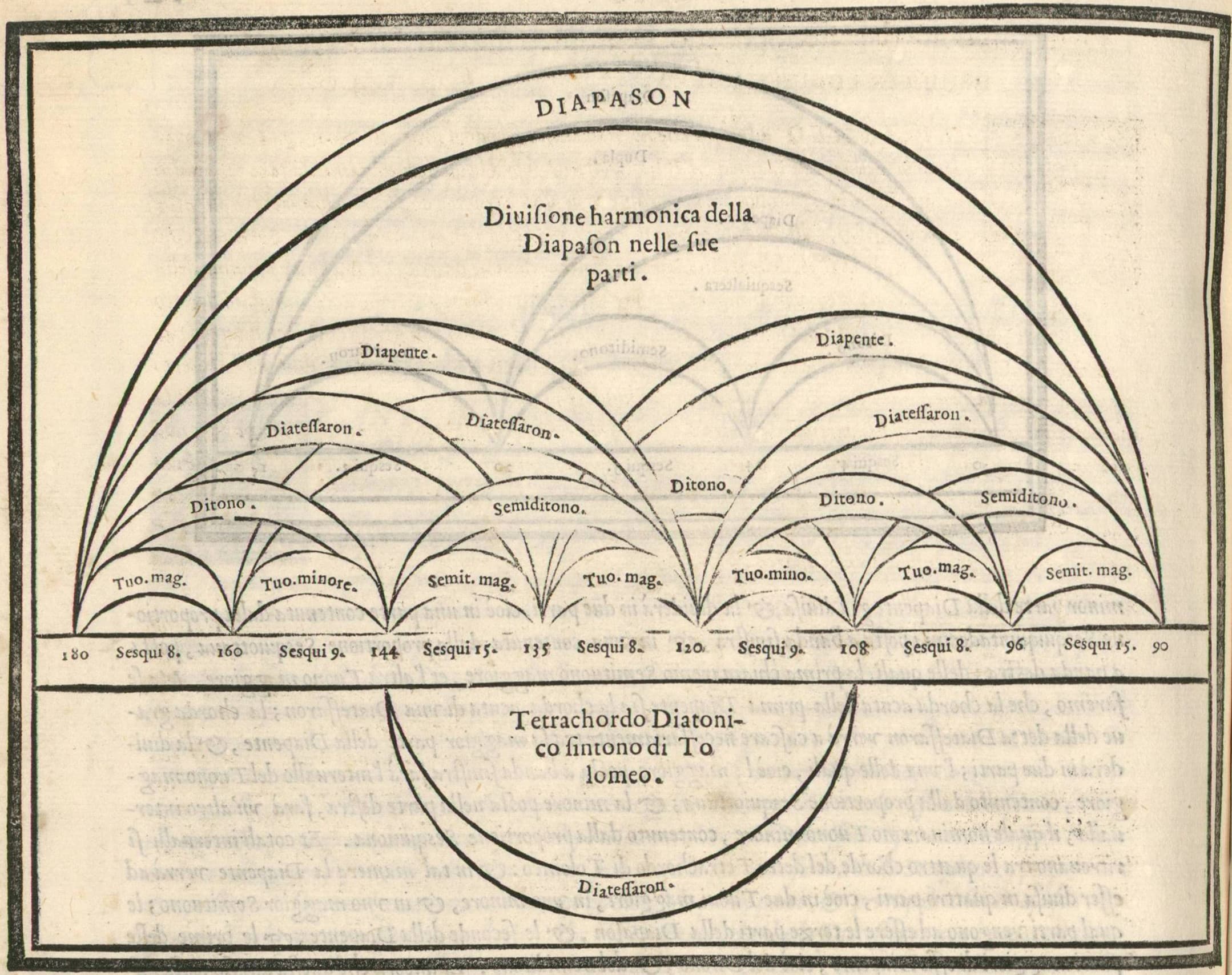
In order to briefly elucidate the musical context of the theorem, we give some examples. Historically, partitions of ratios are frequently written as ordered multi-term proportions within arc diagrams. Arrangements as proportions in lowest terms corresponding to the left-hand side of the following equalities are given in brackets.
All rights reserved.
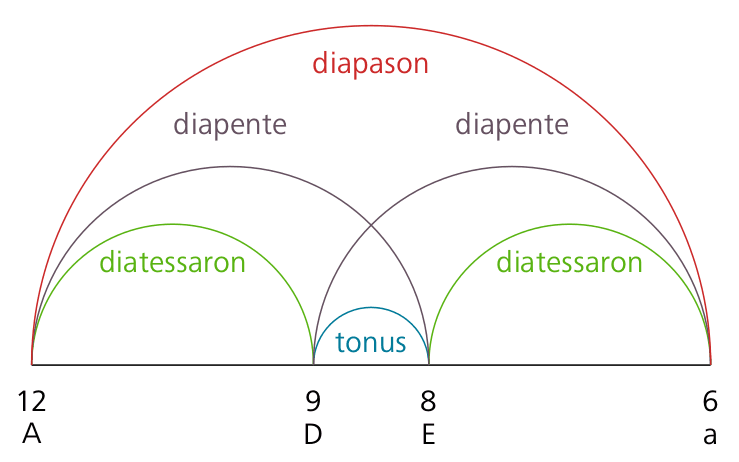
With , the octave (2/1) can be divided into a fifth (3/2) and a fourth (4/3):
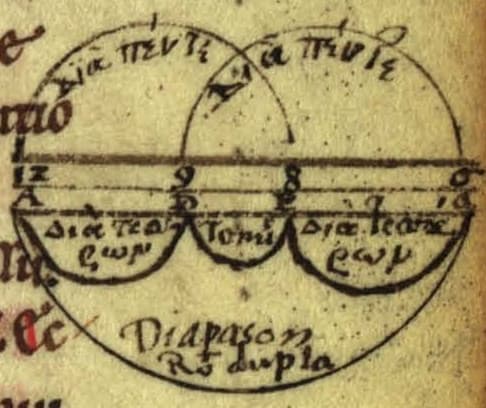
and with , into two fourths and a whole tone (9/8), see Figure 1:
Likewise, the fifth (3/2) can be partitioned with two minor thirds (6/5) and a chromatic semitone (25/24):
However, no epimoric musical interval can be divided into four epimoric smaller intervals, of which three are equal . For example, the Pythagorean division of the fifth into three whole tones and a non-epimoric remainder,
or the division of the octave into three major thirds (5/4) and a diesis (128/125),
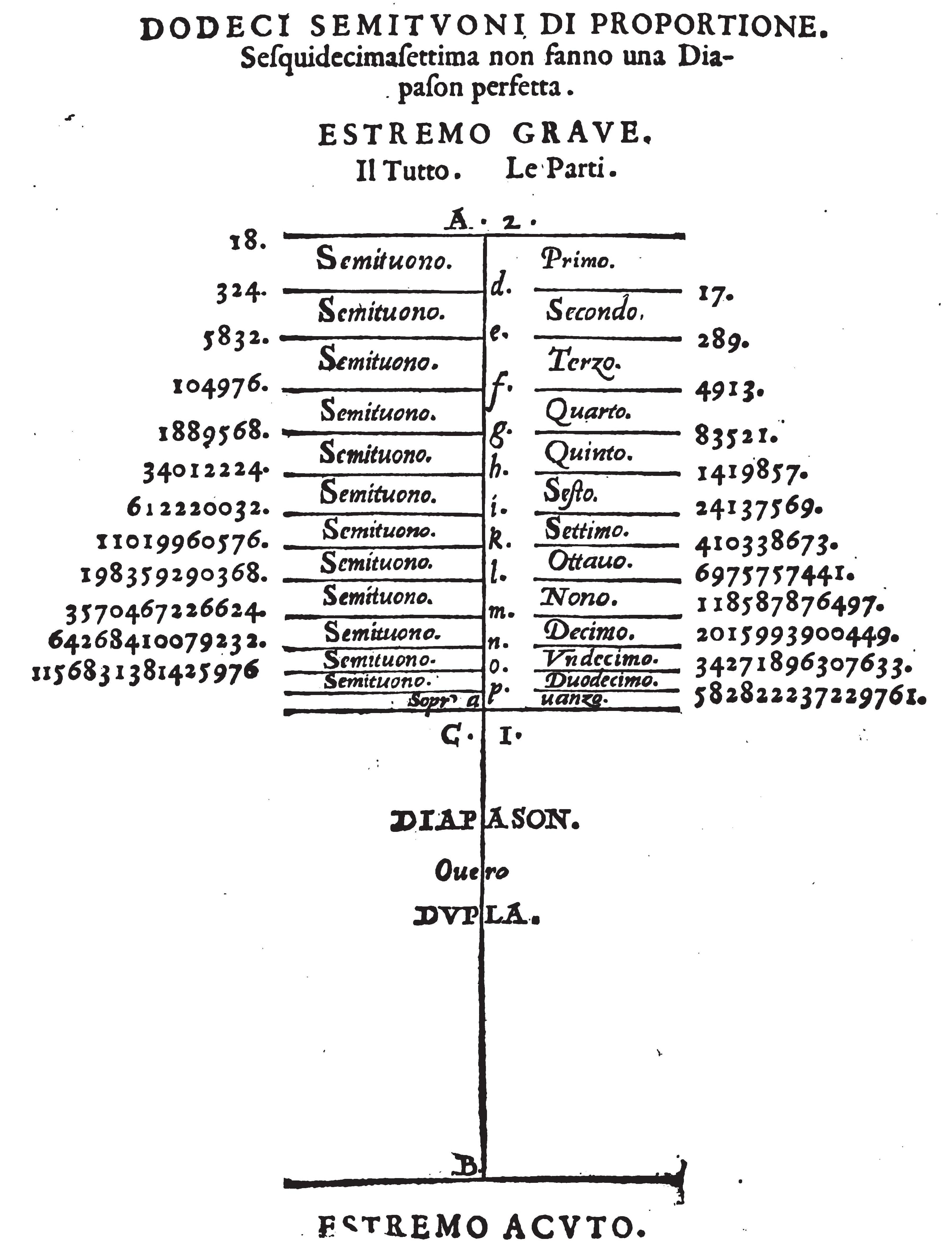
are prototypical: Whatever cubed epimoric ratio is chosen, the cofactors to 3/2 and 2/1 are never epimoric. The latter example illustrates that the just intonation major third (5/4) is an approximation to the problem of doubling the cube, whereas the irrational major thirds of the present-day equal division of the octave is a true solution beyond antique ratio theory8Illustrations for and , where (the octave), from sources of the 14th and 16th century are given in Figure 3..
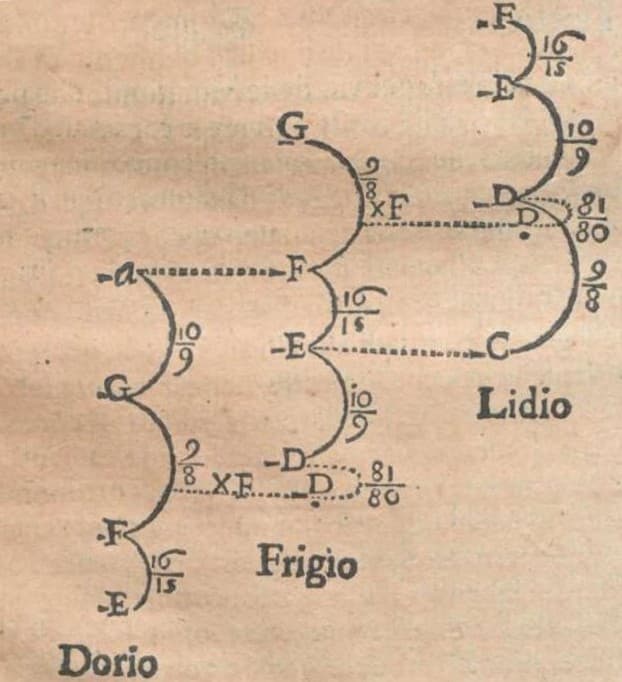
Ptolemy’s tetrachords (divisions of the fourth) involved three different epimoric ratios, as in
Combining this with (12) results in the octave division
where 9/8 and 10/9 define two varieties of whole tones and 16/15 a semitone larger than the Pythagorean 256/243. This partition can be used to define the diatonic scale in just intonation, see Figure 4 (left). The Pythagorean example (13) which fails to be made up solely of epimorics, is an indication for the origin of our problem (1).
3.2 Diophantine equations
Music Theory stood more than once at the origin of fascinating Diophantine equations. For instance, the reputed Catalan equation9Catalan proposed this equation in a French journal in 1841 and it appeared in Crelle’s Journal as a note to the Editor, in 1844., stating that and are the only successive non-trivial powers of integers, generalizes the original question about considered by Philippe de Vitry (1291–1361) in relation with harmonic numbers and Platonic music theory, thus a Diophantine equation with actual connection to music. Levi ben Gershon (1288–1344) had proved that this particular equation does not have other solutions than , and this already in the 13th century. Leonhard Euler (1717–1783) switched exponents and bases in the musical equation, and finally Catalan (1814–1894) allowed both bases and exponents to vary: both latter variations had left the common field of music and mathematics, and Diophantine equations were investigated for their pure mathematical interest.
The present equation (1) still has a lively connection to music theory. Were this of no more concern, one could imagine generalizations of (1) such as
or, defining
one could look at
All this recalls the falling powers
dear to Isaac Newton (1643–1727), and one finds a variant of Fermat’s Last Theorem, that cannot be found among the dozen of variants mentioned in Ribenboim’s 13 Lectures [13 P. Ribenboim, 13 lectures on Fermat’s last theorem. Springer, New York (1979) ], probably the most adequate source for verifying if a variant of Fermat’s Equation has already received attention. This one apparently did not:
We stop here and invite the reader to imagine his own favorite generalization, leaving it to the future to decide whether some of these variations will capture the attention of a larger number of mathematicians, professional or not.
Image sources
Guido of Arezzo (c. 1200), Micrologus, Ms. 8 Cod. Ms. 375 (Cim 13), fol. 53r. Source: München, Universitätsbibliothek
A. M. S. Boethius (early 10th c.), De institutione arithmetica., fol. 4v. Source: Medeltidshandskrift 1 (Mh 1), Lund University Library
Jacobus (Leodiensis) (15th c.), Speculum musicae, Ms. Latin 7207, Vol. III, Cap LXXXV, fol. 46r. Source: gallica.bnf.fr/Bibliothèque nationale de France
G. Zarlino (1588), Sopplimenti musicali, Venetia: Francesco de Franceschi, Sanese, Lib IV, p. 205, https://s9.imslp.org/files/imglnks/usimg/d/d1/IMSLP129044-PMLP252086-terzo_volume.pdf (accessed February 21, 2022)
G. Zarlino (1562), Le istitutioni harmoniche, Venice, Italy, p. 122. https://digital.library.unt.edu/ark:/67531/metadc25955/ (accessed February 21, 2022), University of North Texas Libraries, UNT Digital Library, https://digital.library.unt.edu; crediting UNT Music Library
G. B. Doni (1635), Compendio del Trattato de’ Generi e de’ Modi della Musica, Roma, p. 41. Source: Mus.th. 7234, Bayerische Staatsbibliothek München
- 1
We are aware that the antique ratios are not yet fully fledged rational numbers, i.e., classes of ordered pairs of integers. Whereas ratios were considered equivalent to their representations in lowest terms, the order of the terms of a ratio was not constitutive. The ratios and , for instance, represent the same relationship (the epitriti or sesquitertia) corresponding to the musical interval of the fourth (the diatessaron, see Figure 1) in the sense of a perceptual distance. For our purpose the restriction to rational numbers greater than 1 is sufficient and convenient.
- 2
The term musical interval refers to logarithms of frequency ratios. Many quantifiable sensory phenomena and their physical counterparts are in a logarithmic or nearly logarithmic relationship, as loudness or brightness sensation with respect to the intensity of sound or light.
- 3
The father of Galileo.
- 4
See [1 A. Barker, Greek musical writings: II Harmonic and acoustic theory. Cambridge University Press, Cambridge (1989) , p. 195]; the assignment of the “Sectio Canonis” to Euclid is insecure.
- 5
Irrationality had a precarious ontological status as being defined only ex negativo, and it was linked to incommensurable (geometric) quantities, see also [12 P. Pesic, Hearing the irrational: Music and the development of the modern concept of number. Isis 101, 501–530 (2010) ].
- 6
The conjecture that (1) has no solutions was formulated by the second author, from the study of music theory and on the basis of his mathematical background. It was eventually settled in a joint effort of the two authors.
- 7
This is an other expression for epimoric ratios.
- 8
Illustrations for and , where (the octave), from sources of the 14th and 16th century are given in Figure 3.
- 9
Catalan proposed this equation in a French journal in 1841 and it appeared in Crelle’s Journal as a note to the Editor, in 1844.
References
- A. Barker, Greek musical writings: II Harmonic and acoustic theory. Cambridge University Press, Cambridge (1989)
- A. Barker, Ptolemy’s Pythagoreans, Archytas, and Plato’s conception of mathematics. Phronesis 39, 113–135 (1994)
- A. Barker, Scientific method in Ptolemy’s “Harmonics”. Cambridge University Press, New Haven (2000)
- A. Barker, Mathematical beauty made audible: Musical aesthetics in Ptolemy’s “Harmonics”. Classical Philology 105, 403–420 (2010)
- Boethius, De institutione arithmetica libri duo: De institutione musica libri quinque. B. G. Teubner, Leipzig (1867)
- Boethius, Fundamentals of music. Yale University Press, New Haven (1989)
- P. Gozza, Number to sound: The musical way to the scientific revolution. The Western Ontario Series in Philosophy of Science 64, Springer, Dordrecht (2000)
- G. D. Halsey and E. Hewitt, More on the superparticular ratios in music. Amer. Math. Monthly 79, 1096–1100 (1972)
- C. A. Huffman, Archytas of Tarentum: Pythagorean, philosopher and mathematician king. Cambridge University Press, Cambridge (2007)
- M. Kirnbauer, Vieltönige Musik – Spielarten chromatischer und enharmonischer Musik in Rom in der ersten Häfte des 17. Jahrhunderts. Schola Cantorum Basiliensis Scripta 3, Schwabe, Basel (2013)
- D. Muzzulini, The geometry of musical logarithms. Acta Musicologica 87, 193–216, DOI 10.5281/zenodo.5541790 (2015)
- P. Pesic, Hearing the irrational: Music and the development of the modern concept of number. Isis 101, 501–530 (2010)
- P. Ribenboim, 13 lectures on Fermat’s last theorem. Springer, New York (1979)
- B. V. Rivera, Theory ruled by practice: Zarlino’s reversal of the classical system of proportions. Indiana Theory Review 16, 145–160 (1995)
- F. Sargolini, La critica di Vincenzo Galilei al misticismo numerico di Gioseffo Zarlino. Nuncius 15, DOI 10.1163/182539100X00038 (2000)
- J. Solomon, Ptolemy, “Harmonics”: Translation and commentary. Brill, Leiden (2000)
- S. Stevin, Tafelen van interest, midtgaders de constructie der selver … Christoffel Plantijn, Antwerpen (1582)
- S. Stevin, The principal works of Simon Stevin. Vol. IIA: Mathematics. C. V. Swets & Zeitlinger, Amsterdam (1958)
Cite this article
Preda Mihăilescu, Daniel Muzzulini, A Diophantine equation concerning epimoric ratios. Eur. Math. Soc. Mag. 124 (2022), pp. 16–22
DOI 10.4171/MAG/84