Mathematics at the Deutsches Museum: On-site, digital, and to go

1 Challenge: An abstract science as an exhibition topic

Even the earliest plans for the Deutsches Museum, founded in 1903, included rooms for mathematics. In 1906 the first mathematics exhibition opened in Munich in the former building of the Bavarian National Museum located on Maximilianstraße (today: Museum Fünf Kontinente). In a museum the great challenge to the exhibition makers was, and still is, to identify the topics of mathematics that can be communicated well, as well as to present them to the visitors. Over the decades and with increasing experience, the focus and the way of communication have changed. When the Deutsches Museum was founded, it did not yet have any collections the curators could base the exhibition on. Thus, for the planning of all exhibitions, “lists of desired objects” were prepared by referents. For the mathematics section this was done by Walther von Dyck (1856–1934), mathematician and co-founder of the museum. Based on these wish lists, the collection was first established. The museum received considerable support from various donations and the production of replicas by well-known manufacturers of mathematical instruments and models. After 10 years, the collection of “Mathematische Instrumente, Analoggeräte und -rechner” already counted 500 items. Today it includes more than 4600 objects.

2 Mathematics is fun: The new permanent exhibition “Mathematics”

In July 2022, after nine years of preparation, the Deutsches Museum’s new permanent exhibition “Mathematics” opened (Figure ⁠1⁠).⁠¹⁠More insights on https://www.deutsches-museum.de/museumsinsel/ausstellung/mathematik. Its concept was developed by computer scientist Anja Teuner, who was curator of Computer Science and Mathematics from 2011 to 2018. The hands-on stations, which performed well in the former mathematics exhibition at the Deutsches Museum, the “Mathematisches Kabinett,” were planned to be placed in a larger context and enriched with historical mathematical instruments and models. The intention was to create an exhibition that would satisfy mathematics and at the same time would offer fun, but also provide a place for exhibit lovers to experience and enjoy mathematics.

The exhibition that finally emerged focuses on visualizations of popular geometrical objects and phenomena. A playful approach to mathematics was deliberately chosen – from the descriptive to the abstract. The focus is not on the exhibition objects as such, but rather on active participation, trying things out, and experiencing mathematical structures on one’s own. By these means, mathematics becomes accessible not only for the experienced, but also for the younger visitors and all other interested people. In doing so, the exhibition reduces not only the immense breadth of mathematics, but also the extreme distance that its concepts and ideas have taken from everyday life and vividness. Von Dyck, the first curator of mathematics at the Deutsches Museum, stated as early as 1925: “To present the essence, content, and aims of mathematical research in its entirety cannot be the task of a museum.”⁠²⁠“Wesen, Inhalt und Ziele der mathematischen Forschung in ihrer Gesamtheit vor Augen zu führen, kann nicht Aufgabe eines Museums sein.” [⁠4⁠ W. von Dyck, Mathematik im Museum. In Das Deutsche Museum. Geschichte, Aufgaben, Ziele, C. Matschoss (ed.), VDI-Verlag, Berlin, 192–202 (1925) , p. 192] (translated from German by K. Rasch)

The Mathematics exhibition is based on the formula

The basic elements here are cubes which appear throughout the exhibition in the thematic areas of Introduction, Dimension, Perspective, and Symmetry. “Hanging” cubes contain key information as well as polyhedral interactive media stations, while cube-shaped experiment tables are spread throughout the exhibition. In this way, the interactive concept is placed in a geometric frame of reference, which is complemented by historical exhibits and insights into the practical applications of mathematics. A wide variety of games helps visitors to understand mathematical ways of thinking in a playful way, and richly illustrated exhibition texts reveal how such thinking is put into practice, for example in everyday life or in architecture. Particularly striking exhibits include a wooden pantograph from 1782 for reducing silhouettes, gold-plated multipurpose dividers from 1586, or finely crafted drawing instruments from 1775 made of silver.

From form to formula and back: mathematics makes everything easier – even if a sequence of numbers, letters and symbols may not suggest this at first glance. For example, a shark’s dorsal fin, a triangle in music, and a house gable are just triangles from a mathematical point of view. All triangles have certain common characteristics. These generally valid characteristics then help to create, to comprehend, to describe new items, buildings or technologies. Mathematics – ancient Greek: the “art of learning” – is thus not taught as a fine art, but quite simply as an important aspect of our everyday lives.

3 Gyroid, catenoid, onduloid: Mathematical modelson the Deutsches Museum Digital portal

A relatively short and striking equation:

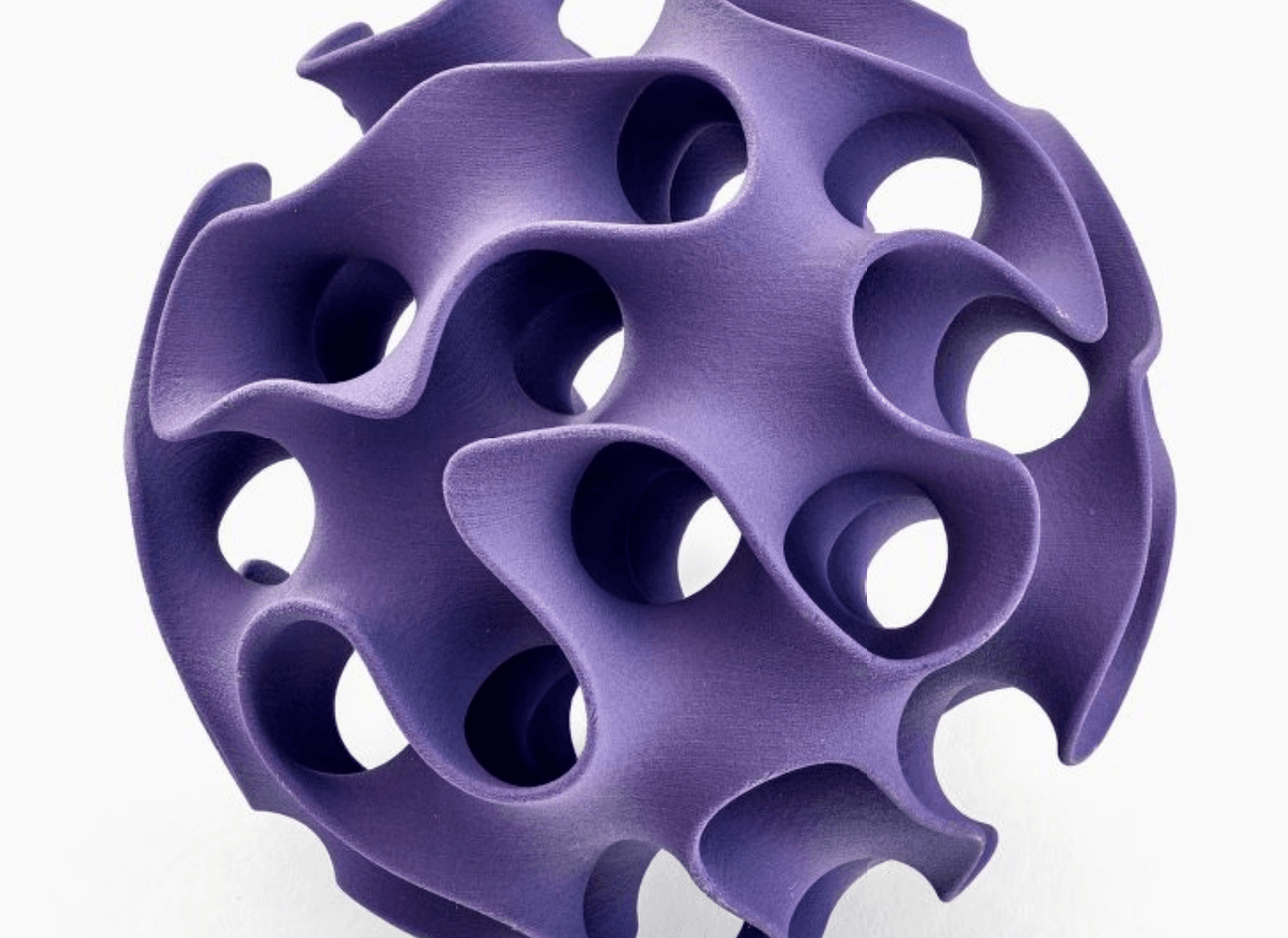
But what does it describe? One recognizes that it contains $x$, $y$ and $z$ and perhaps assumes that a mathematical surface could hide behind the equation. To solve the mystery of what this surface looks like, computer software is used today that makes the surface visible on a screen – or a section of the surface is created using 3-D printing. It can then be inspected from all sides, such as the model of a spherical section of the gyroid⁠³⁠Inv. No. 2018-285, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2018-285 (Figure ⁠2⁠), which was made of purple colored plastic. This model was purchased for the new mathematics exhibition. Once you hold it in your hand, you can imagine more easily that two labyrinths have been interwoven into each other.

Already in the 19th century, there was the wish to visualize mathematical surfaces. At that time, there were no means of digital representation available. People began to construct models of surfaces. Only sections were made, however, since some surfaces extend to infinity. Von Dyck established a collection which was supplemented and expanded over the years and now comprises more than 250 models. Some of them can already be studied in object datasets with high-resolution photos on the online portal Deutsches Museum Digital⁠⁴⁠https://digital.deutsches-museum.de/en in the section “Discover” by clicking on the photo of the gyroid; many more will be added.

The collection of the Deutsches Museum does not only comprise classic models made of plaster, whose prototypes were created in modeling cabinets at universities and were offered for sale in extensive catalogues, such as a catenoid⁠⁵⁠Inv. No. 6449T1, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6449T1 (Figure ⁠3⁠, left) that entered the collection as early as 1906. The museum also preserves fascinating models made of paper or cardboard that change their shape by applying slight pressure, for example an ellipsoid made of 30 cardboard circles⁠⁶⁠Inv. No. 6437, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6437 (Figure ⁠3⁠, middle).

There are also thread and rod models preserved, for example a thread model for the visualization of a hyperboloid of one sheet by its generatrices⁠⁷⁠Inv. No. 6483, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6483 (Figure ⁠3⁠, right), which was produced by the Verlagshandlung Martin Schilling in Halle (Saale) around 1900 based on a design by the mathematician Alexander von Brill (1842–1935) [⁠1⁠ K. Rasch, Fadenmodell zur Darstellung eines einschaligen Hyperboloids. In Die Welt der Technik in 100 Objekten, W. M. Heckl (ed.), C.H. Beck, Munich, 348–353, 671 (2022) ].

Probably worldwide unique are the “women’s tights models” that came to the museum in 2011. The series consists of 42 models, each of which represents a section of a minimal surface. As the sections are extended to infinity in the three spatial dimensions, a surface is obtained that does not intersect itself and divides the space into two congruent labyrinths. Some of these surfaces are used to describe the geometrical arrangement of atoms in particular crystal structures. All the models were laboriously handcrafted from metal wire and women’s tights by the crystallographer Elke Koch in the late 1980s, for example a minimal surface model with a metal base⁠⁸⁠Inv. No. 2012-987T38, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2012-987T38 (Figure ⁠4⁠, left).

Especially difficult to realize are locations in which a surface tapers off to a point. For these singularities, older models had to use supports – as for example this model of a Plücker complex surface (Figure ⁠4⁠, middle). It is made by using painted elements of a lead alloy fixed on a wooden plate. These days, modern manufacturing techniques such as 3-D printing or laser-in-glass technology are used for such complex shapes. The glass models made by mathematician and designer Oliver Labs, such as an Endrass surface⁠⁹⁠Inv. No. 2018-365T4, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2018-365T4 (Figure ⁠4⁠, right), really glow by their bottom illumination.

It is also planned to integrate the mathematical models of the Deutsches Museum into the Digital Archive of Mathematical Models (DAMM), which until now only shows models from university collections.⁠¹⁰⁠https://mathematical-models.org/en

4 Proportional dividers and universal scales: Mathematical instruments on the Deutsches Museum Digital portal

Not only the mathematical models, but all objects of the collection of “Mathematische Instrumente, Analoggeräte und -rechner” are to be successively put online on the Deutsches Museum Digital portal.⁠¹¹⁠https://digital.deutsches-museum.de/en/digital-catalogue/hitlist/?filterCollection=true&filterArchive=false&collection[name]=Mathematische%20Instrumente,%20Analogger%C3%A4te%20und%20-rechner&collection[value]=415 Already today, many scientific instruments such as dividers, slide rules, and planimeters can be studied in detail there with object data, descriptive texts, and object photos that can be enlarged. The latter can be downloaded directly and used under the CC BY-SA 4.0 license. The object data records were enriched with norm data from the Gemeinsame Normdatei (GND) of the Deutsche Nationalbibliothek (for persons and corporate bodies), and with GeoNames (for places), as well as specialist literature, historical sources on instruments, and patents from the German Patent Information System (DEPATISnet). In this way, it is possible to access linked information on several levels if one wants to know more precisely. In addition, the conversion of the object data into the LIDO XML format (developed for the exchange of metadata) will enable the connection to a future national research data infrastructure.

The manufacturers of historical instruments from the early modern period in particular are sometimes more difficult to determine than one might assume at first glance. This fact is shown by the case of a pair of proportional dividers⁠¹²⁠Inv. No. 10505, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/10505 (also: reduction compass) made of gilded copper alloy, probably before 1616 by the clockmaker Heinrich Stolle (Figure ⁠5⁠, left) [⁠7⁠ M. Wöhler, Der Teuffel steckt im Detail: Was Objekte über Personen verraten können. In Mathematik. Vom Anschaulichen zum Abstrakten, K. Rasch (ed.), Verlag des Deutschen Museums, Munich, 220–223 (2023)]. His principal Thomas Ferdinand Teuffel von Zeilberg was previously thought to be the manufacturer. In difficult cases, in addition to knowledge of Latin, so-called historical auxiliary sciences such as heraldry help just as much as the investigation of the object biography and the acquisition history of objects in the archives of the Deutsches Museum, for example in the files of the administrative archives [⁠5⁠ M. Wöhler, Der Gott der Zeit war am schnellsten. Kultur & Technik 4, 56–57 (2019) ]. The search for comparative instruments in other digital object catalogues of museums, such as the catalogues of the British Museum and the Science Museum in London⁠¹³⁠https://collection.sciencemuseumgroup.org.uk andhttps://www.britishmuseum.org/collection, or the catalogues of the Germanisches Nationalmuseum in Nuremberg⁠¹⁴⁠https://objektkatalog.gnm.de/recherche often supports dating and localization of objects. It also makes it possible to assess whether an instrument is singular or whether it was made often – with individual variations or in machine serial production, depending on the century.

Also from a mathematical point of view, the exploiting of objects and their comprehensive explanation for a publication in the digital domain are challenging for museums: A pair of proportional dividers⁠¹⁵⁠Inv. No. 64022, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/64022 (Figure ⁠5⁠, middle) made in Augsburg in 1586 allows geometric operations such as the transfer of line segments as well as the division, multiplication, and transformation of line segments, areas, and bodies as an analog computer [⁠6⁠ M. Wöhler, Multifunktionszirkel. In Die Welt der Technik in 100 Objekten, W. M. Heckl (ed.), C.H. Beck, Munich, 40–45, 662 (2022) ].

The question of processed materials – such as ivory and their plastic surrogates developed since the beginning of the 20th century – is relevant for understanding the objects in terms of their manufacture, users, and contexts of use. However, the materials used do not only determine the price of the object at the time of purchase. Ethical questions about colonial loot and the decimation of animal species also play an increasing role in museum discourse for restitutions and with regard to the resources remaining to humanity in the future. (See the catalogue of the correspondent exhibition in the Humboldt Forum [⁠3⁠ A. Saviello and S. Müller-Wolff (eds.), Terrible beauty: Elephant – human – ivory. Hirmer, Munich (2021) ].) An example of this thematic complex from the mathematical instruments collection is a universal scale⁠¹⁶⁠Inv. No. 1980-182T3, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/1980-182T3 made in England at the beginning of the 20th century (Figure ⁠5⁠, right).

5 Exhibition to go: The new math catalogue

The catalogue accompanying the new permanent exhibition, entitled “Mathematik – Vom Anschaulichen zum Abstrakten,” was recently published by the museum’s own publishing house [⁠2⁠ K. Rasch, Mathematik – Vom Anschaulichen zum Abstrakten. Deutsches Museum Verlag, Munich (2023) ]. The visual approach to mathematics taken in the exhibition is continued here. At the same time, exhibit lovers can enjoy a wide range of finely crafted drawing instruments, delicate surface models and precise mathematical instruments. In addition, numerous essays and thematic pages illuminate the diversity – and beauty – of mathematics from different angles, thus complementing the exhibition. Many models, dividers and other types of instruments can be studied in these more profound texts and in the catalogue section and can be compared with one’s own prior knowledge or other collections.

In addition to interested readers of the catalogue, we are looking forward to welcome expert visitors to the new permanent exhibition. The new museum app also provides relevant background information in advance and, if desired, accompanies you in a relaxing manner through the exhibitions.⁠¹⁷⁠Free download for Android and Apple via https://www.deutsches-museum.de/en/museum-island/visit/app-audioguide.

Katja Rasch is curator of mathematics and is responsible for the collection of mathematical instruments and analog computing devices at the Deutsches Museum. She is currently working on the digital catalogue of the collection of mathematical models. The mathematician has been a research associate at the Deutsches Museum since 2015. Previously a mathematics teacher and working for a schoolbook publisher, one of her main areas of research work is the didactics of mathematics. k.rasch@deutsches-museum.de Mareike Wöhler has been a research associate at the Research Institute of the Deutsches Museum since 2017. The historian works in the Deutsches Museum Digital team on the digital catalogue and exploration of the object collection (including mathematical instruments, time measurement, navigation, and geodesy). She is concerned with the manufacturers, production, and history of objects for measuring time and space under the overarching cultural-historical question of why objects of everyday life and objects of knowledge have changed concretely over the centuries. m.woehler@deutsches-museum.de

1. ¹

   More insights on https://www.deutsches-museum.de/museumsinsel/ausstellung/mathematik.

2. ²

   “Wesen, Inhalt und Ziele der mathematischen Forschung in ihrer Gesamtheit vor Augen zu führen, kann nicht Aufgabe eines Museums sein.” [⁠4⁠ W. von Dyck, Mathematik im Museum. In Das Deutsche Museum. Geschichte, Aufgaben, Ziele, C. Matschoss (ed.), VDI-Verlag, Berlin, 192–202 (1925) , p. 192] (translated from German by K. Rasch)

3. ³

   Inv. No. 2018-285, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2018-285

4. ⁴

   https://digital.deutsches-museum.de/en

5. ⁵

   Inv. No. 6449T1, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6449T1

6. ⁶

   Inv. No. 6437, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6437

7. ⁷

   Inv. No. 6483, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/6483

8. ⁸

   Inv. No. 2012-987T38, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2012-987T38

9. ⁹

   Inv. No. 2018-365T4, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/2018-365T4

10. ¹⁰

    https://mathematical-models.org/en

11. ¹¹

    https://digital.deutsches-museum.de/en/digital-catalogue/hitlist/?filterCollection=true&filterArchive=false&collection[name]=Mathematische%20Instrumente,%20Analogger%C3%A4te%20und%20-rechner&collection[value]=415

12. ¹²

    Inv. No. 10505, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/10505

13. ¹³

    https://collection.sciencemuseumgroup.org.uk andhttps://www.britishmuseum.org/collection

14. ¹⁴

    https://objektkatalog.gnm.de/recherche

15. ¹⁵

    Inv. No. 64022, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/64022

16. ¹⁶

    Inv. No. 1980-182T3, https://digital.deutsches-museum.de/en/digital-catalogue/collection-object/1980-182T3

17. ¹⁷

    Free download for Android and Apple via https://www.deutsches-museum.de/en/museum-island/visit/app-audioguide.

References

1. K. Rasch, Fadenmodell zur Darstellung eines einschaligen Hyperboloids. In Die Welt der Technik in 100 Objekten, W. M. Heckl (ed.), C.H. Beck, Munich, 348–353, 671 (2022)
2. K. Rasch, Mathematik – Vom Anschaulichen zum Abstrakten. Deutsches Museum Verlag, Munich (2023)
3. A. Saviello and S. Müller-Wolff (eds.), Terrible beauty: Elephant – human – ivory. Hirmer, Munich (2021)
4. W. von Dyck, Mathematik im Museum. In Das Deutsche Museum. Geschichte, Aufgaben, Ziele, C. Matschoss (ed.), VDI-Verlag, Berlin, 192–202 (1925)
5. M. Wöhler, Der Gott der Zeit war am schnellsten. Kultur & Technik 4, 56–57 (2019)
6. M. Wöhler, Multifunktionszirkel. In Die Welt der Technik in 100 Objekten, W. M. Heckl (ed.), C.H. Beck, Munich, 40–45, 662 (2022)
7. M. Wöhler, Der Teuffel steckt im Detail: Was Objekte über Personen verraten können. In Mathematik. Vom Anschaulichen zum Abstrakten, K. Rasch (ed.), Verlag des Deutschen Museums, Munich, 220–223 (2023)